Motor Driving Two Gears

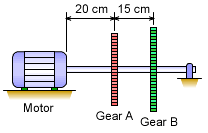
It has been determined that a mechanical drive system needs to generate 900 N-m torque (clockwise) to turn two gears. Gears A and B have resisting moments of 500 N-m and 400 N-m respectively (counter-clockwise which is the opposite direction to the motor moment). The shaft needs to be sized to insure the safe operation of the system.

What is known:

• The drive shaft is straight and continuous from the motor to the right support.
• The shaft material is an aluminum alloy with E = 69 GPa and ν = 0.33.
• The maximum shear stress of the shaft is 275 MPa.
• A factor of safety of 2.0 is required.
• The rpm of the motor is not known.

Considering the factor of safety, what is the minimum radius so that the shear stress does not exceed the given stress limit? What is the total shaft rotation from one end to the other?

• The motor torque must equal the moment loads applied to the shaft from the gears.
• The shaft internal moment needs to be determined (may not equal the gear loads).
• To determine the required radius, use the torsional shear stress equation,
  
       
  
• After the radius is found, use the angle of twist equation to find the rotation angle,