| Ch 1. Stress and Strain | Multimedia Engineering Mechanics | ||||||
|
Normal Stress |
Shear and Bearing Stress |
Normal Strain |
Hooke's Law |
Thermal Effects |
Indeterminate Structures |
||
| Thermal Effects | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
MECHANICS - THEORY |
||||||||||||||||||||
| Thermal Strain |
||||||||||||||||||||
for Common Materials (Expanded List in Appendix) |
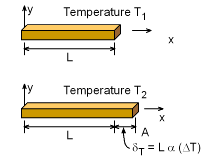
When physical materials are heated, they generally expand due to atomic-level changes. This expansion is proportional to the change in temperature. In terms of strain, this relationship is written as
where α is the coefficient of thermal expansion. This constant is different for all materials and is generally a positive number. There are a few materials that contract when heated, and then this constant would be negative. The coefficient of thermal expansion represents a quantity (i.e. strain) per degree C or per degree F. |
|||||||||||||||||||
| Thermal Expansion |
||||||||||||||||||||
|
|
The total deflection of a member that undergoes a temperature change, ΔT = T2 - T1, can also be written as
The deflection is in the direction of the length L. For a 3D homogenous object, thermal expansion will occur in all three directions. The total deflection in any given direction will be a function of the length, width or depth of the object. There is no shear strain or shear deflection due to thermal expansion. |
|||||||||||||||||||
| Combined Thermal and Mechanical Strain or Deflection |
||||||||||||||||||||
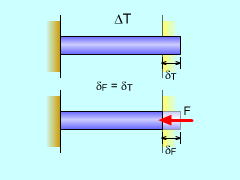 Rod Between Two Fixed Walls - Example of Stresses induced by Thermal Expansion |
Generally, thermal expansion is accompanied with mechanical deflection caused by a physical load. When both force and temperature changes are present, special care is needed when solving the problem. Both the thermal and mechanical deflections need to be compatible. For example, if a simple rod is placed between two fixed walls and heated, the rod will try to expand. However, the walls will prohibit the normal thermal expansion and induce a compression force. This force is real and causes the rod to decrease in length. The final result is the thermal expansion is offset by the mechanical compression deflection (see diagram and animation at left). When both mechanical and thermal conditions are present, a compatibility relationship needs to be introduced to solve the problem. Further discussion of compatibility conditions can be found in the following section on Indeterminate Axial Structures. |
|||||||||||||||||||
| Other Material Properties |
||||||||||||||||||||
|
|
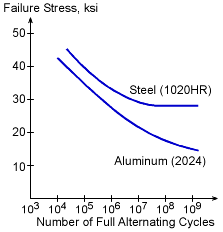
Cyclic Loading (Fatigue) As structural members experience multiple loading/unloading, their failure stress level will decrease. For example, car axles or even aircraft wings will experience millions of loadings over their service life. For aluminum, the failure stress can reduce by 70% of the original failure stress level. To assist designers, charts called s-n curves are developed through experiments. The charts show the failure stress changes as the object is subjected to cycles. In some industries, such as aircraft, extensive testing on full scale models are required to insure the structure will not failure before a certain number of load cycles. |
|||||||||||||||||||
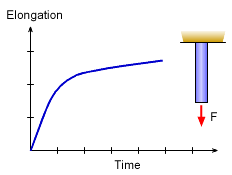 Typical Creep Curve |
Viscoelasticity (Creep) Another interesting property of many materials, especially plastics, is viscoelasticity or creep. This property models a material's tendency to flow over time when under stress. The most common effect of this is the deformation of an object over time when under a very low load. Even glass will creep over time. Plastics tend to exhibit higher creep characteristics then metals or ceramics.
|
|||||||||||||||||||
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.