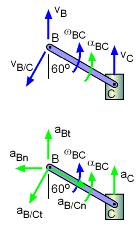
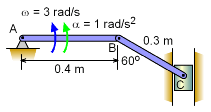
Two bars link a vertically sliding piston, C, to a pinned point, A. If bar AB rotates at a rate of 3 rad/s and accelerates at a rate of 1 m/s², what is the angular acceleration of bar BC?

Velocity and Acceleration Diagrams

Velocity information about bar BC must first be found before accelerations can be determined. The key object in this system is rod BC. Velocity of points B can be found, and velocity direction of C can be found.

Velocity
First, find the velocity of point B by relating its velocity to fixed point A,
     v_B = v_A + v_B/A = 0 + ω_AB × r_AB
          = 3k × 0.40i = 1.2j m/s

The velocity direction of C is known, thus
     v_c = v_cj m/s

Combining gives,
     v_B = v_C + v_B/C = v_C + ω_BC× r_BC
     1.2j = v_Cj + ω_BCk × (-0.3 sin60i + 0.3 cos60j)
     1.2j = v_Cj + 0.2598ω_BCj + 0.15ω_BCi
     i terms:  ω_BC = 0

Acceleration
Now the acceleration terms can be determined since the velocity information has been calculated. The acceleration of point B is,
     a_B = a_A + a_A/B
         = a_A + ω_AB² r_AB + α_AB × r_AB
         = 0 + (3)² (0.4i) +1k × 0.4i
         = 3.6i + 0.4j

Acceleration of point C,
     a_C = a_cj m/s²

Combining,
     a_B = a_C + a_B/C
     a_B = a_C + ω_BC² r_BC + α_BC × r_BC
     3.6i + 0.4j = a_Cj + 0 +
                  + a_BCk × (-0.3 sin60i + 0.3 cos60j)
     3.6i + 0.4j = a_Cj + 0.2598 a_BCj + 0.15α_BCi
     i terms:   -3.6 = -0.15 α_BC

α_BC = 24 rad/s²