| Ch 8. Internal Loads | Multimedia Engineering Statics | ||||||
|
Int. Forces and Moments |
V and M Diagrams I | V and M Diagrams II | V, M, Load Relationships | ||||
| Shear, Moment and Load Relations | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
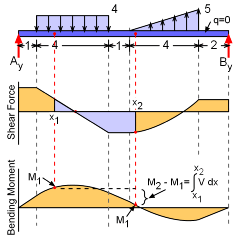
| In the previous two sections, methods where presented to construct shear and moment diagrams. However, there are some important relationships between loading, shear and moment which can aid in the construction of the diagrams. | ||
|
|
Relationship between Shear Force and Distributed Loads |
|
|
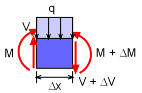
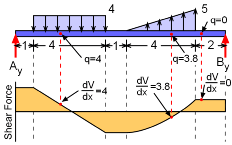
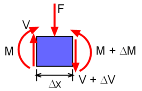
For a distributed load, q, the forces can be summed in the y direction to give, ΣFy = V - (V + ΔV) - q Δx = 0 This can be rearrange to give, ΔV/Δx = -q If the limit of both sides is taken as Δx goes to zero, then the relationship between shear force and distributed loads becomes dV/dx = -q This can also be written in integral form as Graphical examples of both these equations are shown at the left. |
||
|
|
Relationship between Bending Moment and Shear Force |
|
|
Similar to the shear force in the previous paragraphs, the moments can be summed for a beam element with a distributed load of "q", giving, ΣM = -M + (M+ ΔM)
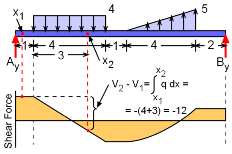
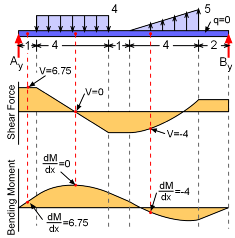
This equation can be rearranged to give, ΔM/Δx = V + ΔV + q (Δx/2) Using the previous relationship for ΔV gives ΔM/Δx = V - q Δx + q (Δx/2) Now, take the limit of both sides as Δx goes to zero. Last term vanishes and a direct relationship be between bending moment and shear force is identified as, dM/dx = V This can also be written as an integral relationship, Two examples using the above equations are shown at the left. |
||
|
|
Effect of a Point Load |
|
|
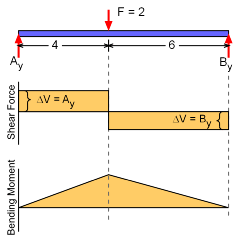
For a beam element subjected to a point load, the forces can be summed in the vertical to give, ΣFy = V - (V + ΔV) - F = 0 ΔV = -F This represents that point loading will cause a jump in the shear diagram equal to the point load. The moment will not jump with a point load. |
||
|
|
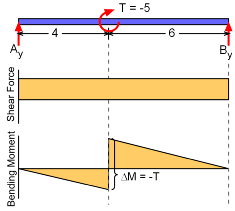
Effect of a Point Moment |
|
|
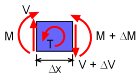
For a beam element subjected to a point moment as shown, the shear force at that point is unaffected; but if the moments are summed about the left edge, then ΣM = ΔM - (V + ΔV)Δx - T = 0 If the limit as Δx goes to zero is taken, then it becomes ΔM = -T
|
||
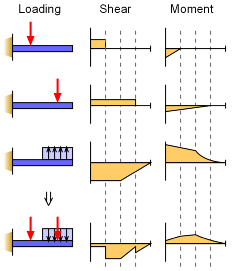 Principle of Superposition |
Principle of Superposition |
|
|
For beams with several different loads, it is often easier to solve for the shear and moment diagrams for the individual loads and then combine them to find the total shear and moment diagrams. This is known as the method, or principle, of superposition. For the cantilevered beam shown, shear and moment diagrams can be constructed for the two point loads and for the distributed load, separately; they can then be combined to form the full shear and moment diagrams.
|
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.