| Ch 4. Beam Stresses | Multimedia Engineering Mechanics | ||||||
|
Bending Strain and Stress |
Beam Design |
Shear Stress |
Built-up Beams |
||||
| Built-up Beams | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Example |
||
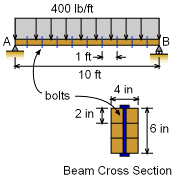 Bolted Beam Constructed from Parts |
A simply support beam is constructed from 3 long board, each 4 inches wide by 2 inches thick. The boards are held together with bolts that are evenly spaced at 1 ft intervals. What minimum bolt diameter must be used so that the shear stress in any bolt is not more than 10 ksi. Note that there are bolts at the ends (points A and B). |
|
| Solution |
||
|
|
Like with most mechanics problems, the boundary or support reaction should be determined. Then from that, a simple shear diagram can be constructed which will help find the maximum shear at any point in the beam. |
|
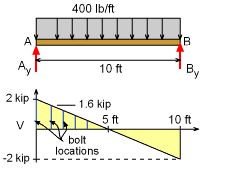 Shear Diagram |
Ay = By = 400(10)/2 = 2 kip Since the load is a uniform distributed load across the whole beam, the shear diagram will vary linearly from one end to the other, as shown in the diagram. The shear diagram indicates that the maximum shear force in the beam is at either end of 2 kip. However, the end bolt will only have to carry half the shear stress between the end bolt and the next bolt. On the other hand, the second bolt will need to carry shear stress over a larger distance of the beam. |
|
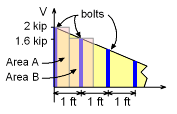 Shear Load Carried by First Two Bolts |
The actual shear load carried by each bolt is better understood by looking at a diagram that shows how the shear stress between bolts is distributed to the first two bolts. Area A in the diagram is carried by the first bolt. Even though the shear force magnitude is higher, the second bolt will have to carry more of the actual shear stress in the the beam. Thus, the shear force, V, in beam at the location of the second bolt is V = 4/5 (2 kip) = 1.6 kip Thus, 1.6 kip will be used to find the average shear stress at the location of the second bolt. The shear stress is found by using, τ = VQ / Ib |
|
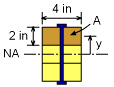 Determining Q for Given Location |
The other terms in this equation are, I = 634/12 = 72 in4 The shear stress at the location of the second bolt is τ = (1.6)(16)/[(72)(4)] = 0.088889 ksi |
|
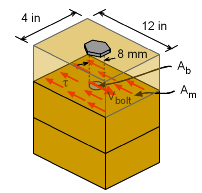 Shear Load Carried by First Two Bolts |
This stress acts between the top board and the next board as shown in the diagram on the left. The total shear load between the boards that must be carried by the second bolt is, Vbolt = Am τ The shear stress in the bolt is The bolt must have a cross-sectional area of Ab = 0.4266 in2 This requires a radius of r = (Ab/π)0.5 = (0.4266 π)0.5 = 0.3684 or d = 0.7368 in The nearest nominal size would be 3/4 in. |
|
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.