| Ch 1. Stress and Strain | Multimedia Engineering Mechanics | ||||||
|
Normal Stress |
Shear and Bearing Stress |
Normal Strain |
Hooke's Law |
Thermal Effects |
Indeterminate Structures |
||
| Normal Stress | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Example |
||
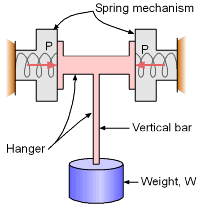 Spring Load Hanging Bracket |
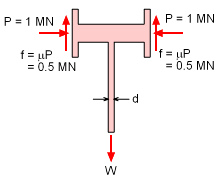
A "quick release" hanger is designed to carry a weight by using friction. The hanger is suspended by using two spring mechanisms that exert a constant horizontal force P of 1 MN on the two sides of the hanger. The vertical bar that holds the weight is circular and has negligible weight. What is the minimum diameter of this circular bar that can carry the maximum load W? Maximum allowable stress, σ, for the vertical bar material is 100 GPa and coefficient of friction, μ, between sides of hanger and rough surface of spring mechanism is 0.5. |
|
| Solution |
||
|
|
The spring mechanisms exert a horizontal force P of 1 MN. This force generates friction, f at both sides of the hanger. f = μP Since, the whole hanger system is suspended only by this frictional force, it can carry a maximum weight, W of W = 2f = 2(0.5) = 1 MN Ignoring the weight of the hanger, the minimum cross sectional area, A, of the vertical part, is σ = F/A Corresponding diameter of the rod, |
|
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.