| Ch 10. Vibrations | Multimedia Engineering Dynamics | ||||||
| Free Vibs. Undamped | Free Vibs. Damped | Forced Vibration | Energy Method | ||||
| Free Vibrations- Undamped | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
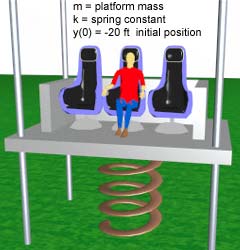
A free-body diagram can be used to identify all the forces on a the platform. The weight, mg, will act on the spring but the spring force will counter-act the weight, so the weight actually cancels. The spring deflection will cause a force, ky, that will accelerate the platform. The final equation of motion becomes, The general solution of this differential equation is y(t) = A sinωnt + B cosωnt where ωn represents the natural frequency and is defined as |
|
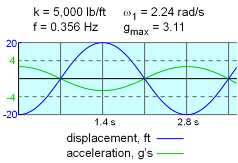
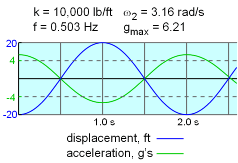
For the two cases, k1 = 5,000 and k2 = 10,000, the natural frequencies are found: case 1: ωn1 = 2.24 rad/s case 2: ωn2 = 3.16 rad/s The initial conditions for this particular situation are y(0) = -20 ft These two conditions can be used to determined the constants A and B. The final equation becomes y(t) = -20 cosωnt |
||
|
The velocity equation is determined by taking the time derivative of the displacement equation, dy/dt = -20 ωn sinωnt Likewise, the acceleration equation is found taking the second time derivative of the displacement, d2y/dt2 = -20 ωn2 cosωnt Therefore, the maximum acceleration is directly related to the square of the natural frequency, or This can be converted to g forces by dividing by the acceleration of gravity, gmax = 20/32.2 ωn2 = 0.621ωn2 g's The motion of the platform and its acceleration are plotted at the left for the two spring constants of 5,000 and 10,000 lb/ft. The 10,000 lb/ft case is unacceptable since the acceleration exceeds the safety limit of 4 g's. |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.