| Ch 5. Rigid Body General Motion | Multimedia Engineering Dynamics | ||||||
| Fixed Axis Rotation | Plane Motion Velocities | Zero Velocity Point | Plane Motion Accelerations |
Multiple Gears |
Rot. Coord. Velocities | Rot. Coord. Acceleration | |
| Multiple Gears | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
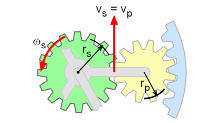
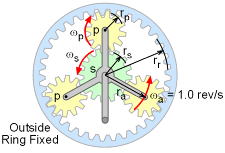
The objective in designing the salad spinner is to maximize the spin rate of the inside bowl (connect to the sun gear) for a given handle (or arm) angular velocity. The epicyclical gear mechanism has four different objects that can rotate. For the salad spinner, the outside ring gear is fixed and the arm or handle angular velocity is given as 1 rev/s. This leaves two other gears with unknown angular velocities, the sun and planet gears. Since the handle mechanism rotates about its center and its angular velocity is known, the linear velocity of the arm tip, va, is va = ωa ra However, this is also the linear velocity of the planet gear center. Because the ring gear does not rotate, the velocity at the point of contact between the planet gear and the ring gear is zero (outside edge). By using similar triangles, the edge velocity of the planet gear where it contacts the sun gear, vp, can be calculated as vp = 2 va = 2(ωa ra) |
|
|
\
|
Since the the planet gear and the sun gear are in contact (and do not slip), their tangent linear velocities must be equal, giving vs = vp Since the sun gear is pinned, vs = rsωs. Substituting for vs and vp gives ωsrs = 2 ωa ra ωs = 2 ωa ra/rs The angular velocity magnification is dependent on the ratio of the carrier radius to the sun gear radius, ra/rs . A small sun gear and a large planet gear will produce the highest rotation rate for the bowl. Thus, the best radius for the sun gear ra is 0.5 inch (remember, there are only 4 gear sizes available). This requires the planet gear radius to be 1.5 inches. ωa = 2 (1 rev/s) 1.5 in / 0.5 in = 6 rev/s This gives a speed magnification of 6 to 1. |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.
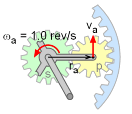
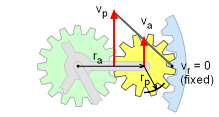 Inside Velocity of Planet Gear
Inside Velocity of Planet Gear