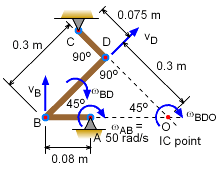
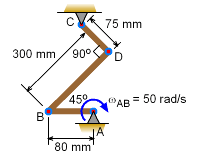
The rod AB rotates at ω_AB= 50 rad/s about the fixed point A. The rod, CD is pinned at point C. Determine the angular velocity of the rod CD at the instant shown.

Point of Zero-Velocity Method

This could be solved using relative motion equations (vectors) as explained previous section, General Plane Motion: Velocities. However, for many velocity plane motion problems, using the instantaneous center of zero velocity (IC) can simplify the problem.

The IC point will be at the intersection of the two lines perpendicular to the velocities of point B and D. For this instant, the triangle OBD is rotating about point O.

The distances DO and BO are,
     r_OD = r_BD tan45 = 0.3 (1) = 0.3 m
     r_OB = r_BD / cos45 = 0.3 / 0.7071 = 0.4243 m

The velocity of point B is,
      v_B = ω_AB r_AB = 50 (0.08) = 4 m/s

Thus, the angular velocity of BD is,
     ω_BD = ω_BDO = v_B/r_BO = 4 / 0.4243 = 9.428 rad/s

The velocity of point D can now be determined,
     v_B = ω_BD r_OD = 9.428 (0.3) = 2.828 mr/s

Finally, the angular velocity of CD is
     ω_CD = v_B / r_CD = 2.828 / 0.075 = 37.71

ω_CD = 37.71 rad/s