| Ch 3. Forces & Particle Equilibrium | Multimedia Engineering Statics | ||||||
|
Equilibrium and FBD |
2-D Forces |
3-D Forces |
|||||
| 2-D Forces (point) | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
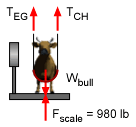
| Part a) - Bull's Actual Weight |
||
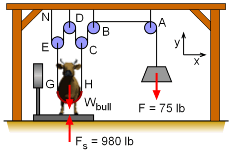 Problem Layout with Given Information
|
A good place to start for all statics problems is a free-body diagram (FBD). However, in this problem, because there are numerous objects, it can be confusing which object (or objects) to use in a FBD. Since the bull seems to be central to the problem, it would be a good place to start (note, there is no "wrong" place to start). The FBD of the bull is shown at the left. Summing forces in the y-direction gives, ΣFy = Fscale + TCH + TEG - Wbull = 0 Wbull = 980 lb + TCH + TEG The rope tensions TCH and TEG must be equal since it is assumed that the sling holding the bull is symmetrical. This gives, Wbull = 980 lb + 2TCH (1) However,
you will notice that there are still two unknowns, Tch and
Wbull. More information is needed. |
|
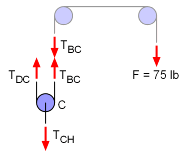 Pulley E and D Diagrams |
The next logical object to examine is pulley C since it is connected to the bull. Its FBD is shown at the left along with the rope connected to the counterweight. Summing forces on pulley C gives, ΣFy = TBC + TDC - TCH = 0 The rope must have the same tension since the pulleys are assumed mass-less, TBC = TDC = F = 75 lb The rope tension TCH can be calculated as, TCH = 150 lb Substitute TEG and TCH into Eq. 1 to find the
weight of the bull, |
|
| Part b) - Counterweight |
||
|
In part a), the bull's weight was found. Now, the problem is reversed, the bull weight is known, 1,368 lb, and the counterweight needs to be determined to make the scale register 1000 lb. |
||
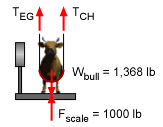 FBD of Cow for Part b) |
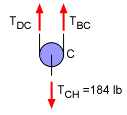
This time it is easier to start with the bull and work though the pulleys to find the counterweight. The FBD for the bull is shown at the left. Summing forces in the y-direction gives the tension in the ropes TEG and TCH, ΣFy = Fscale + TCH + TEG - Wbull = 0 TCH + TEG = 368 lb It can be assumed (as done in part a) that the bull's weight is equally distributed between the two ropes holding the cow. Thus TCH equals TEG giving, TCH = TEG = 368/2 = 184 lb |
|
|
The next step is to analyze the pulley C since the tension is rope CH is known. The FBD of the pulley is given at the left. Summing forces gives, ΣFy = TBC + TDC + TCH = 0 The tensions TBC and TDC are equal, TBC = 184/2 = 92 lb Since the rope has the same tension as it goes from pulley C to the counterweight, F = TFA = TAB = TBC The magnitude of the counterweight needed to just register 1000 lb on the scale is F = 92 lb |
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.