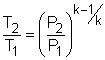A leak develops in a well-insulated bottle initially containing compressed carbon dioxide. The mass of carbon dioxide left in the bottle needs to be determined.

Assumptions:

• No significant heat transfer occurs.
• Since the carbon dioxide leaks slowly, the process is assumed reversible.
• The carbon dioxide is modeled as an ideal gas with constant specific heats.

Take the mass of carbon dioxide
remaining in the bottle after
leak as a system

The Process on the T-s Diagram

Molar Mass, Gas Constant for Various Common Gases

Specific Heats of Some
Common Ideal Gases

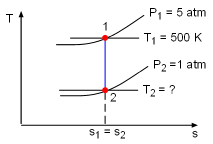
Take the bottle as the system. Denote the state before leak as state 1 and the state after leak as state 2.

From the assumptions made, carbon dioxide is modeled as an idea gas. With the ideal-gas equation of state, the mass remains in the bottle after leak is

      m₂ = P₂V₂/RT₂

where P₂ and V₂ are known. If the temperature T₂ is determined, the mass remaining m₂ can be obtained. The gas constant R of carbon dioxide is 188.9 J(kg-K).

The leaking process is adiabatic and reversible, hence it is an isentropic process. The second relation of isentropic process for ideal gases is:

Assume the final temperature is 350 K and use the average value of the initial temperature and the final temperature to determine the value of k.

      T_av = (500 + 350 )/2 = 425 K

From the table,  k equals to 1.246 at 425 K.

With all the data known, T₂ can be obtained by substituting the values of T₁, P₁, and P₂ into the above equation.

      T₂ = 500(1/5)^0.246/1.246 = 363.9 K

The calculated final temperature is close to 350 K (the assumed temperature), hence no iteration is needed.

Inserting T₂, P₂, and V₂ into the expression for m₂ gives,

      m₂ = (101,325)(200/1,000)/((188.9)(363.9))
           = 295 g