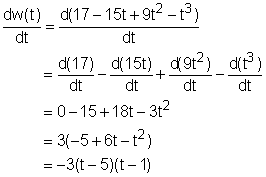| Ch 4. The Mean Value Theorem | Multimedia Engineering Math | ||||||
|
Maximum & Minimum |
Rolle's Theorem |
Mean Value Theorem |
Monotonic Functions |
First Derivative Test |
Concavity & Inflection |
Second Derivative Test |
|
| Monotonic Functions | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| MATHEMATICS - CASE STUDY SOLUTION |
|||||||||||||||||||||||||
 Work Function |
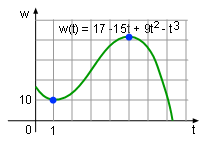
Determining whether the expression, w(t) = 17 - 15t + 9t2 - t3, is the work function of an liver implant assist device. The power should be positive for this liver implant assist device to work because the device is consuming power to generate energy. Recall that the derivative of the work function with respect to time is power, and thus the way to check whether the function is the proper work expression of this device is to figure out the sign of the power, in other words, the monotonicity of the work function. Overall, the derivative of the work expression needs to be calculated which will give an expression for power. That is The critical point where the maximum and minimum energy may occur can be calculated by setting dw/dt to 0. -3(t - 5)(t - 1) = 0 Solving this equation gives t = 1 or t = 5 It is clear that the signs of the power, dw/dt, depends on two factors, t - 1 and t - 5. The domain of time is between 0 and +∞, which is broken down to three intervals by 1 and 5, they are (0, 1), (1, 5), and (5, +∞). The sign and the monotonicity are shown in the table. A "+" indicates a positive expression and a "-" means a negative expression. |
||||||||||||||||||||||||
|
|||||||||||||||||||||||||
| According to the table, the interval for this device to work properly is between 1 and 5 years because in this period of time the sign for dw/dt is positive and the function is increasing. This makes no sense in the real life because the device does not work for the first year, but works for the following four years. Therefore, the expression is not the work function for the liver assist implant device. | |||||||||||||||||||||||||