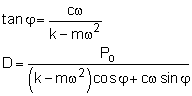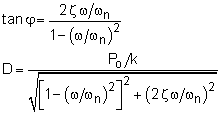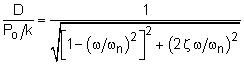| Ch 10. Vibrations | Multimedia Engineering Dynamics | ||||||
| Free Vibs. Undamped | Free Vibs. Damped | Forced Vibration | Energy Method | ||||
| Forced Vibrations | Case Intro | Theory | Case Solution |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||||||
|
|
Recall, for a spring-mass-damper system (damped) under free vibration, the general equation of motion is m d2y/dt2 + c dy/dt + ky = 0 Similarly, for horizontal motion, |
|||||
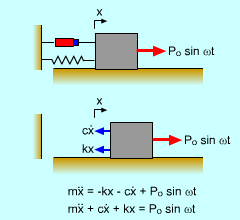 Development of Motion Equation |
However, it is common for a damped system to have an external harmonic force acting on the system. For example, unbalanced rotating motors will cause sever forces on the mounting brackets. This system is called forced vibrations. A forced vibration is one in which the system is excited by an external, time-varying force P, called a forcing function. In this case In general, forcing functions are periodic, and since any periodic function can be expressed as a Fourier series, it is convenient to look at a forcing function of the type P(t) = Posinωt or P(t) = Pocosωt Considering the sin term, the differential equation is The solution of this differential equations consists of two parts, the complementary or homogenous solution, x(t) = xc(t) + xP(t) |
|||||
The complementary solution is the general solution of the homogeneous equation as presented in the damped free vibrations section. Recall, for an underdamped system, the solution is Since the forcing function is sin, the particular solution will be of the form xP(t) = Dsin(ωt - φ) Substituting the particular solution into the differential equation and take the appropriate derivatives gives D[(k-mω2)cosφ + cω sinφ] sinωt The sin and cos terms can be separated to get the two equations D[(k-mω2)cosφ + cω sinφ] = Posinωt D[cω cosφ - (k-mω2)sinφ] = 0 Now the constants, D and φ, can be obtained as |
||||||
The D and φ terms can also be written using ωn and ζ terms, giving
|
||||||
To better understand the solution, it is convenient to consider the ration of the static deflection δP, to the dynamic deflection, D. This ratio can be calculated as
A plot for several damping ratios, ζ, is shown below. Notice that when the external force frequency is close to the system natural frequency, systems with low damping have large displacement amplitudes. This is commonly known as resonance. The total solution for the underdamped case is where the constants A and B must be determined from the initial conditions. |
||||||
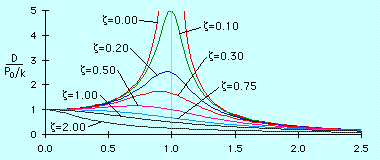 Effect of Various Damping Factors |
||||||
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.