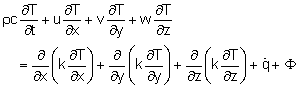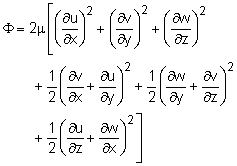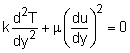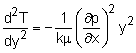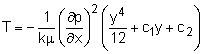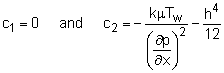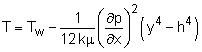The energy equation in differential form will be presented in this section. The details of the derivation are omitted, with attention focused on the use of the equation.

As discussed in the Conservation of Energy section, the energy equation is derived based on the first law of thermodynamics, which states that energy can neither be created nor destroyed; it can only change form. Similar to the derivations applied to derive the continuity and Navier-Stokes equations, the energy equation can be obtained by applying the first law of thermodynamics on a small differential fluid element. The derivation details are omitted for simplicity, and the energy equation for an incompressible fluid is given by

where c is the specific heat, T is the temperature, k is the thermal conductivity, is the rate of internal heat generation (e.g., chemical, electrical and nuclear energy) within the fluid, and Φ is the dissipation function due to the viscous forces.

The energy equation expressed in terms of Cartesian coordinates is

where the viscous dissipation function is given by

As mentioned in the Moment of Momentum section section, there are three heat transfer modes: heat conduction, heat convection and radiation. The left hand side of the energy equation represents the local and convective terms, while the first three terms on the right represent the conductive terms. From the energy equation, it is noted that in order to solve for the temperature distribution, the velocity distribution is needed, which makes the analysis complex and tedious. Often, numerical methods such as finite difference and finite element methods are used to tackle these problems. However, analytical solutions do exist for some specific cases, and one such example is discussed next.

Flow between Fixed Parallel Plates

Velocity Profile

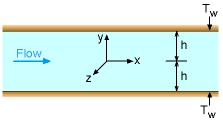
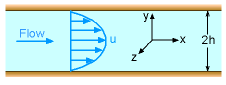
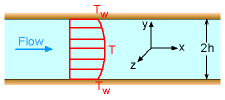
Consider steady, incompressible, laminar flow between two infinite parallel horizontal plates as shown in the figure. The velocity profile is found to be,

where ∂p/∂x is treated as a constant and represents the pressure drop along the channel length. The velocity profile is parabolic, and it is a function of y only (as shown in the figure). Suppose that both plates are maintained at the wall temperature T_w, the temperature profile of the fluid can then be obtained from the energy equation. Assume that the plates are wide enough such that the temperature variation is only in the y-direction. The steady-state energy equation reduces to

where the thermal conductivity is assumed constant.

Substitute du/dy from the obtained velocity profile into the energy equation and rearrange terms to yield

The above equation can then be integrated twice to obtain

where the constants c₁ and c₂ can be determined from the temperature boundary conditions (i.e., T = T_w at y = h and -h) such that

The temperature profile is thus given by

     

Note that due to the viscous dissipation, the temperature of the fluid inside the channel is actually higher than the wall temperature.