Ch 3. Fluid Kinematics     Multimedia Engineering Fluids
Flow
Descriptions		 Steady &
Unsteady		 Streamlines,
Streaklines		 Velocity &
Acceleration		 Irrotational
Flow		      
Fluids
 Flow Descriptions Case Intro Theory Case Solution  
 Chapter
 1. Basics
 2. Fluid Statics
 3. Kinematics
 4. Laws (Integral)
 5. Laws (Diff.)
 6. Modeling/Similitude
 7. Inviscid
 8. Viscous
 9. External Flow
 10. Open-Channel
 
 Appendix
 Basic Math
 Units
 Basic Equations
 Water/Air Tables
 Sections
 
Search
 
 eBooks
 Dynamics
 Fluids
 Math
 Mechanics
 Statics
 Thermodynamics
 
Author(s):
Chean Chin Ngo
Kurt Gramoll
 
©Kurt Gramoll
FLUID MECHANICS - CASE STUDY SOLUTION

 

  

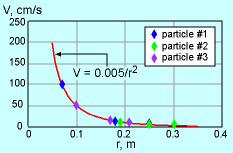
Since it is given that the velocity is inversely proportional to the square of the location r, assume the correlation for velocity has the following form:

     V = c/r2

where c is a constant which needs to be determined.
     

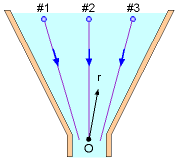
Schematic of Experiment Set #1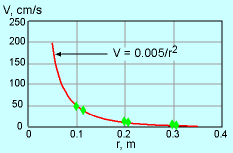


Correlation for Experiment Set #1		  

For experiment set #1, the locations of the measurement points are first determined (using the Pythagorean theorem):

Sensor Location A B C
Location (r), m 0.304 0.300 0.304
Velocity (V), cm/s 5.2 5.6 5.5
c = Vr2, m3/s 0.00481 0.00504 0.00508
Sensor Location D E F
Location (r), m 0.206 0.200 0.206
Velocity (V), cm/s 11.5 12.4 11.7
c = Vr2, m3/s 0.00488 0.00496 0.00497
Sensor Location G H I
Location (r), m 0.112 0.100 0.112
Velocity (V), cm/s 40.0 50.5 39.5
c = Vr2, m3/s 0.00502 0.00505 0.00495

By taking the average of the calculated values for the constant c, it is found that c is 0.00497, which is close to 0.005. The Eulerian viewpoint is used in this experiment since the velocities are measured at fixed locations.
     


Schematic of Experiment Set #2


Correlation for Experiment Set #2

  

For experiment set #2:

Fluid Particle #1
Location (r), m 0.25 0.18 0.07
Velocity (V), cm/s 8.1 15.5 101.8
c = Vr2, m3/s 0.00506 0.00502 0.00499
Fluid Particle #2
Location (r), m 0.30 0.25 0.19
Velocity (V), cm/s 5.7 7.8 14.0
c = Vr2, m3/s 0.00513 0.00488 0.00505
Fluid Particle #3
Location (r), m 0.21 0.17 0.10
Velocity (V), cm/s 11.0 16.9 50.0
c = Vr2, m3/s 0.00485 0.00488 0.00500

The average value for the constant c is 0.00498, which is again close to 0.005. Since the measurements in set #2 are taken by following individual fluid particles, the flow field is determined using the Lagrangian viewpoint.
     
   
 
Practice Homework and Test problems now available in the 'Eng Fluids' mobile app
Includes over 250 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.