| Ch 2. Particle Force and Acceleration | Multimedia Engineering Dynamics | ||||||
|
Rect. Coord. |
Normal/Tang. Coord. |
Polar Coord. |
Orbital Mechanics | Computational Mechanics | |||
| Normal/Tangential Coordinates | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
|||||
|
|
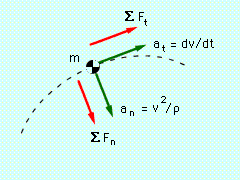
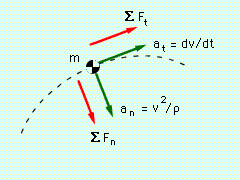
Previously, in the Curvilinear Motion: Normal/Tangential Coordinates section in chapter 1, equations for the normal and tangential components of acceleration were derived for a particle moving in a plane curved path. These were, at = dv/dt an = v2/ρ Newton's Second Law can also be expressed in terms of normal and tangential coordinates, ΣFtet + ΣFnen = m (atet + anen ) Substituting gives, ΣFtet + ΣFnen = m (dv/dtet + v2/ρen ) Or for just the tangent direction, ΣFt = m dv/dt In the normal direction, ΣFn = m v2/ρ |
||||
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.