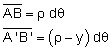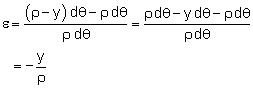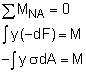| Ch 4. Beam Stresses | Multimedia Engineering Mechanics | ||||||
|
Bending Strain and Stress |
Beam Design |
Shear Stress |
Built-up Beams |
||||
| Bending Strain and Stress | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
|||||
|
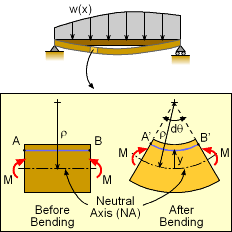
Knowing the shear and moment at any location in a beam is only useful if that information can be used to design a beam. The shear and moment need to be used to determine the stresses which can be used to find if the material will fail This section will examine bending stress and how it can be calculated from the bending moment. The following section will consider shear stress. |
|||||
| Beam Bending Strain |
|||||
|
|
To understand the bending stress in an arbitrary loaded beam, consider a small element cut from the beam as shown in the diagram at the left. The beam type or actual loads does not effect the derivation of bending strain equation. Recall, the basic definition of normal strain is ε = ΔL/L Using the line segment, AB, the before and after length can be used to give The line length AB is the same for all locations before bending. However, the length A'B' becomes shorter above the neutral axis (for positive moment) and longer below. The line AB and A'B' can be described using the radius of curvature, ρ, and the differential angle, dθ. Notice that the y coordinate is assumed upward from the neutral axis, where there is no strain. Putting these together gives, This relationship gives the bending strain at any location as a function of the beam curvature and the distance from the neutral axis. However, this equation is of little use, and needs to be converted to stress. Also, radius of curvature is difficult to determine at a given beam location. |
||||
| Beam Bending Stress |
|||||
|
|
The strain equation above can be converted to stress by using Hooke's law, σ = Eε, giving, σ = -Ey/ρ (1) There is still the issue of not knowing the radius of curvature, ρ. If one thinks about it, the radius of curvature and the bending moment should be related. |
||||
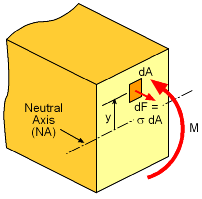 Beam Section Cut |
This relationship can be determined by summing the moment due to the normal stresses on an arbitrary beam cross section and equating it to the applied internal moment. This is the same as applying the moment equilibrium equation about the neutral axis (NA). For a positive moment, the top stresses will be in compression (negative stress) and the bottom stresses will be in tension (positive stress) and thus the negative sign in the equation. This equation can be changed by using equation (1), It is interesting to note that the integral is the area moment of inertia, I, or the second moment of the area. Many handbooks list the moment of inertia of common shapes (see Sections appendix). A review of moment of inertia is given below in the next sub-section. Using the area moment of inertia gives E I / ρ = M |
||||
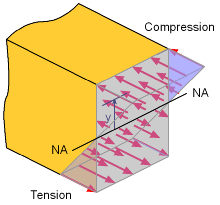 Bending Stress Distribution |
But the radius of curvature, ρ, is still there. But equation (1), ρ = -Ey/σ , can be used again to eliminate ρ, giving, E I /(-Ey/σ ) = M Simplifying and rearranging gives,
This equation gives the bending normal stress, and is also commonly called the flexure formula. The y term is the distance from the neutral axis (up is positive). The I term is the moment of inertia about the neutral axis. |
||||
| Locating the Neutral Axis |
|||||
|
|
If the cross section is symmetrical about the horizontal axis, then the neutral axis is halfway between the top and bottom. However, for non-symmetrical beam, such as a "T" cross section, the neutral axis is not halfway between the top and bottom, and needs to be determined before the bending stress equation can be used. |
||||
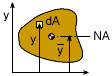 Centroid for Arbitrary Shape |
The neutral axis is located at the centroid (geometric center) of the cross section. Recall from Statics, the centroid can be found using two methods. The first is by integration,
|
||||
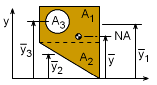 Centroid Based on Sub-shapes |
The second, and more common method, is the method of parts. The beam cross section is split into geometric shapes that are common (rectangle, triangle, circle, etc.). The centroid of basic shapes can be found in handbooks (see Appendix Sections), eliminating the need for integration. The centroid is
If there is a hole, then that area is considered to be negative, and the same equation can still be used. As an example, the diagram at the left would be, |
||||
| Area Moment of Inertia |
|||||
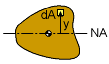 Moment of Inertia around Neutral Axis using Integration |
Similar to the centroid, the area moment of inertia can be found by either integration or by parts. The moment of inertia is also called the "second moment of the area" since that describes the integration equation, When using this with the bending stress equation, I is about the neutral axis and not the x-axis. |
||||
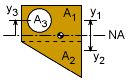 Moment of Inertia around the Neutral Axis using Parts |
A more common method to find the moment of inertia is by parts. Like finding the centroid (needs to be done first), the object is split into smaller basic shapes. The moment of inertia about the centroid of each part can be found in a handbook (see Appendix Sections). Then the individual moment of inertia's are moved to the neutral axis using the parallel axis theorem. For a particular sub-shape, this gives
where Ii is the moment of inertia about its own shape and INA-i is the moment of inertia about the object's neutral axis. All the moment of inertia terms can then be added together to give, INA = Σ INA-i For the diagram at the left, the parts method gives, I = (I1 + y12 A1) + (I2 + y22 A2) - (I3 + y32 A3) Notice, for a hole, the moment of inertia is subtracted for that shape. |
||||
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.