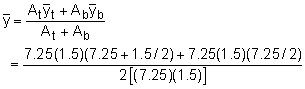| Ch 4. Beam Stresses | Multimedia Engineering Mechanics | ||||||
|
Bending Strain and Stress |
Beam Design |
Shear Stress |
Built-up Beams |
||||
| Bending Strain and Stress | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
When designing structures, many times a dimension needs to be determined. In this problem, the load and beam cross section is given, but its length is not. This is the main concern since the length also determines the moment. This problem can be solved by first finding the moment in terms of the unknown length L. Then determine the moment of inertia. Finally, use the bending stress equation and solve for the length. |
|
| Maximum Moment |
||
|
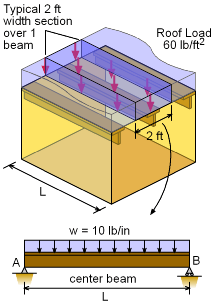
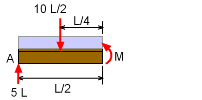
Since the bending stress depends on the moment, it needs to be determined. The center beams carry the roof load over a two foot width of the roof as shown in the diagram. The total load on the beam in terms of inches will be, w = (60 lb/ft2)(2 ft)(1 ft/12 in) = 10 lb/in This constant distributed load will cause a maximum moment at the center (this can be determined by constructing a moment diagram, but has been omitted here). Cutting the beam at the center and summing moments gives the moment equation, ΣMright edge= 0 M - 5L(L/2) + [10 (L/2)] (L/4) = 0 M = 1.25 L2 lb-in As expected, it is a function of the unknown length L. |
||
| Moment of Inertia |
||
|
|
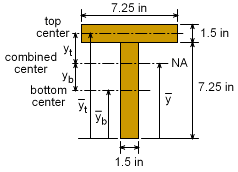
The moment of inertia needs to be found for the complete beam cross section, but this shape is not in any handbook. To simplify finding the moment of inertia, the beam can be separated into two rectangular shapes, giving, Itop = h3b/12 = 1.53 (7.25) / 12 = 2.0391 in4 Ibottom = h3b/12 = 7.253 (1.5) / 12 = 47.635 in4 Using the parallel axis theorem, the moment of inertia for both of these can be found at the centroid of the actual "T" beam. But first, the center needs to be located. The vertical centroid is Now that the center is known, the moment about the NA can be determined as INA = It + At yt2 + Ib + Ab yb2 = 2.039 + 7.25(1.5)(7.25
+ 0.75 - 5.8125)2 + = 2.039 + 52.04 + 47.63 + 52.04 = 153.7 in4 |
|
| Bending Stress |
||
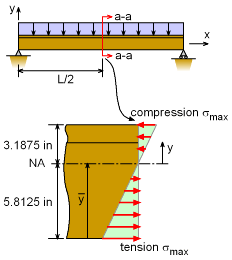 Beam Bending Stresses at Section a-a: Positive Moment Causes Compression at top and Tension at Bottom. |
The material bending stress cannot exceed 7,500 psi. and there is also a factor of safety of 2.0. Using the bending stress equation gives, σ = -My/I 7,500/2.0 = -(1.25 L2)(-y) / 153.7 The y distance is the furthest from the neutral axis. For this T shaped beam, it would be in the tension zone, with y = -5.8125 in. 576,400 = 1.25 L2 (5.8125) Solving for L gives L = 281.7 in = 23.47 ft It is interesting to note, that if just a single 2x8 is used in the vertical position (I = 47.63, y = 3.25), then the span would be limited to 16.55 ft. Thus, even with the moment of inertia over three times larger for the T-beam, the span is not even double. This is due to the squared length term in the moment. |
|
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.