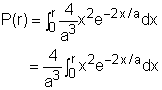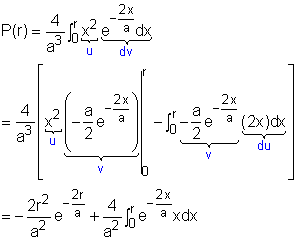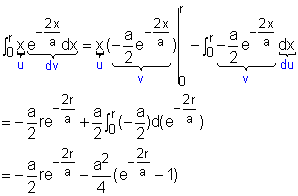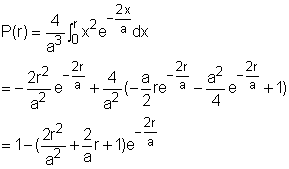| Ch 8. Integration Techniques | Multimedia Engineering Math | ||||||
|
Integration by Parts |
Trig. Integrals Trig. Subst. |
Fractions & Substitutions |
Approximate Integration |
||||
| Integration by Parts | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
The probability that the electron will be found within a spherical volume of radius 3a needs to be determined ( a is a constant). |
||
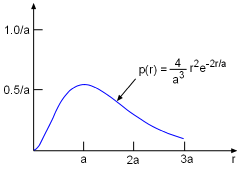 The Probability density Function p(r) |
The probability function is: The integration by parts formula is given as To integrate this function, Consider u = x2 and
|
|
|
||
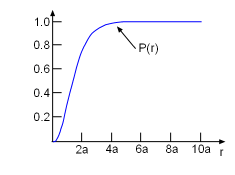 The Probability Function P(r) |
Use integration by parts again to determine the second term of the above expression. Let u = x and dv = e-2x/adx, then du = dx and Substituting this expression into the expression of P(r) gives Therefore, That means the electron has 93.8% chance to be found within a sphere of 3a radius. |
|