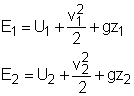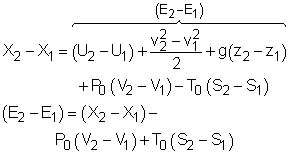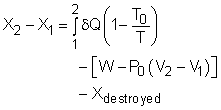| Ch 7. Exergy Analysis | Multimedia Engineering Thermodynamics | ||||||
| Exergy |
Exergy Change |
Exergy Balance(1) |
Exergy Balance(2) |
||||
| Exergy Balance (1) | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Pure Substances |
| 3. First Law |
| 4. Energy Analysis |
| 5. Second Law |
| 6. Entropy |
| 7. Exergy Analysis |
| 8. Gas Power Cyc |
| 9. Brayton Cycle |
| 10. Rankine Cycle |
| Appendix |
| Basic Math |
| Units |
| Thermo Tables |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
| Exergy Balance for a Closed System
|
||
Exergy balance for a closed system can be developed by combining the energy and entropy balances for a closed system. When a closed system undergoes a process from state 1 to state 2, its energy and entropy balances are
Energy Balance:
Multiplying the second equation by T0 and subtracting it from the first one yields,
|
||
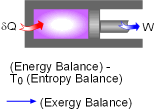 A Closed System |
A closed system contains internal, kinetic, and potential energies. At state 1 and state 2, the total energy in a closed system is, The exergy of the mass in the closed system at state 1 and state 2 are: Subtracting X1 from X2 gives, Replacing (E2 - E1) in equation (1) with the above equation, and rearranging it gives, |
|
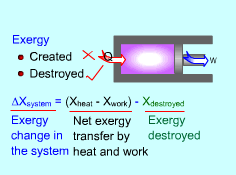 Exergy Balance for Closed System |
The above expression is the exergy balance of a closed system undergoing a process from state 1 to state 2. The terms on the right hand are:
There is a third term in the exergy balance because exergy can not be created, but only can be destroyed. Therefore, the exergy change of a system equals the difference between the net exergy transfer through the system boundary and the exergy destroyed in the system. For a closed system, exergy transfer through the system boundary is due to exergy transfers by heat and work. |
|


 (1)
(1)