| Ch 4. The Mean Value Theorem | Multimedia Engineering Math | ||||||
|
Maximum & Minimum |
Rolle's Theorem |
Mean Value Theorem |
Monotonic Functions |
First Derivative Test |
Concavity & Inflection |
Second Derivative Test |
|
| The Mean Value Theorem | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| MATHEMATICS - CASE STUDY SOLUTION |
||
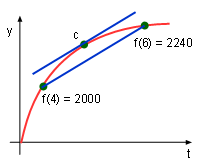 Driving Process (Unit: mile) |
Peter is driving on on a road where the speed limit is
65 mi/hr. By 4 p.m. he has drove 2000 miles and by 6 p.m. his total trip
mileage goes up to 2240 miles. Was Peter speeding during in this period
of time? By 4 p.m. he traveled 2000 miles, so f(4) = 2000 By 6 p.m. he traveled 2240 miles, so f(6) = 2240 The mean value theorem gives that In this case, the mean value on interval [4, 6] is df(c)/dx = (f(6) - f(4))/(6 - 4) = (2240 - 2000)/2 = 120 Since f(t) is a position function, the derivative of it is velocity. In other words, the above equation means that there is a time c in the interval of 4 and 6 such that the velocity is 120 mi/hr. Therefore, Peter exceeded the speed limit. |
|