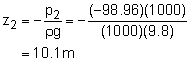| Ch 7. Incompressible and Inviscid Flow | Multimedia Engineering Fluids | ||||||
|
Bernoulli's Equation |
Flow Measurements |
Superposition of Flows |
Flow around Cylinder |
||||
| Bernoulli's Equation | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Fluid Statics |
| 3. Kinematics |
| 4. Laws (Integral) |
| 5. Laws (Diff.) |
| 6. Modeling/Similitude |
| 7. Inviscid |
| 8. Viscous |
| 9. External Flow |
| 10. Open-Channel |
| Appendix |
| Basic Math |
| Units |
| Basic Fluid Eqs |
| Water/Air Tables |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
|
|
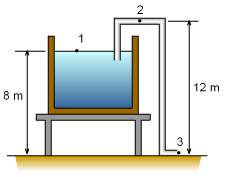
Applying Bernoulli's equation between points 1 and 2 yields For a large open tank, p1 = 0 (gage pressure) and V1 = 0. Rearranging the above equation to gives Thus the pressure at point 2 can be determined once the velocity at point 2 (V2) is known. To determine V2, apply Bernoulli's equation between points 1 and 3, and recognize that the velocity at points 2 and 3 is equal for constant diameter pipe and a steady rate of flow. The pipe outlet acts as a free jet, so p3 = 0 (gage pressure), and z3 = 0 (datum). Thus the velocity at point 2 is then given by The gage pressure at point 2 thus can be simplified as, p2 = -0.5ρ (2gz1) + ρg (z1 -
z2) To avoid cavitation (i.e., p2 cannot be less than the vapor pressure), the maximum height of z2 should be |
|
Practice Homework and Test problems now available in the 'Eng Fluids' mobile app
Includes over 250 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.