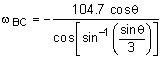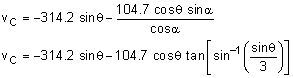| Ch 5. Rigid Body General Motion | Multimedia Engineering Dynamics | ||||||
| Fixed Axis Rotation | Plane Motion Velocities | Zero Velocity Point | Plane Motion Accelerations |
Multiple Gears |
Rot. Coord. Velocities | Rot. Coord. Acceleration | |
| General Plane Motion: Velocities | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
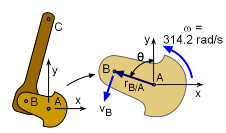
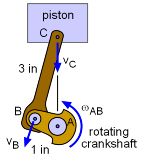 Piston Configuration Labels |
This piston problem can be solved by breaking the problem into two parts.
After the velocity of point C is known in terms of the rotation angle θ, the problem could be optimized to find the maximum velocity. |
|
| Part a) Motion of Bar AB |
||
|
|
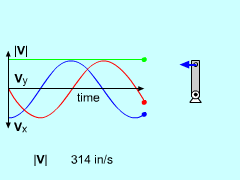
Use the relative velocity equation to find the velocity of B. Note that point A does not translate. vB = vA + vB/A = 0 + ωAB × rB/A Next, the angular velocity, ω = 3,000 rpm, needs to be converted into radians per second. 3000 rpm (1 min/60 s) (2π/1 rev) = 314.2 rad/s The relative distance vector now needs to be defined. Referring to the diagram, we get rB/A = -|rB/A| sinθi + |rB/A| cosθj = -1" sinθi + 1" cosθj = -sinθi + cosθj Substituting this into the relative velocity equation above gives the final velocity of point B in terms of the angle θ. The angle θ is still not known but will be determined when the maximum velocity is found. vB = 314.2k × ( -sinθi + cosθj ) = -314.2 cosθi - 314.2 sinθj in/s |
|
| Part b) Motion of Bar BC |
||
|
|
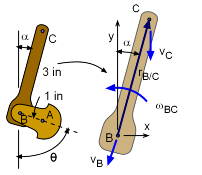
Once again, the relative velocity equation is used to determine the velocity of a point on a rotating and translating bar. vC = vB + vC/B = vB + ωBC × rC/B The velocity of point B is known from previous analysis and the velocity of point C is constrained in the vertical direction. The angular velocity ωBC is not known. vCj = -
314.2 cosθi - 314.2 sinθj + Completing the cross product and equating the i and j components gives, i: 0 = -314.2 cosθ - 3ωBC cosα j: vC = -314.2 sinθ + 3ωBC sinα The two angles θ and α are actually related (see diagram). The horizontal distance of both arms must equal each other since points A and C always aligned in the vertical direction. (3 in) sinα = (1 in) sinθ Substituting α = sin-1 (sinθ /3) into the i terms and solving for ωBC gives, gives This can now be used with the j terms to solve for the velocity of C in terms of the angle θ, |
|
| Finding Maximum Velocity of Point C |
||
|
|
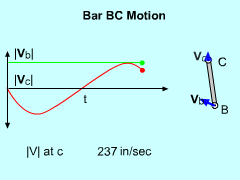
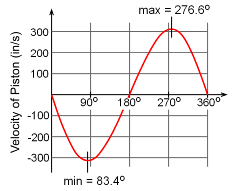
Up to this point, the velocity vC has been derived for any angle θ. The angle that gives the maximum velocity now needs to be determined. This can be done by taking a derivative with respect to the angle θ and equating it to zero. Without the help of a symbolic math program such as Mathcad™ or Mathematica™, closed-form derivation becomes nearly impossible. Even then, the result is complex. The final solution, using numerical methods, for the maximum velocity of point C is θ = 1.455 rad = 83.4o vmax = +/- 316.3 in/s The velocity curve is plotted for point C in the diagram at the left. |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.