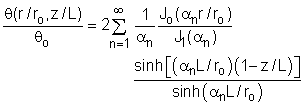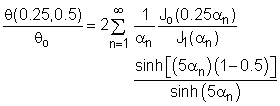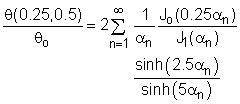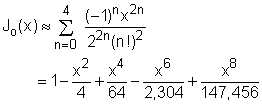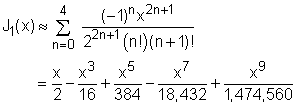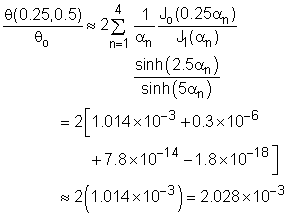Ch 12. Infinite Sequences and Series     Multimedia Engineering Math
Sequences
and Series		 Integral and
Comparison		 Alt. Series &
Abs. Conv.		 Power
Series		 Taylor, Mac. &
Binomial		      
Math
 Power Series Case Intro Theory Case Solution  
 Chapter
 1. Limits
 2. Derivatives I
 3. Derivatives II
 4. Mean Value
 5. Curve Sketching
 6. Integrals
 7. Inverse Functions
 8. Integration Tech.
 9. Integrate App.
 10. Parametric Eqs.
 11. Polar Coord.
 12. Series
 
 Appendix
 Basic Math
 Units
 
 eBooks
 Dynamics
 Fluids
 Math
 Mechanics
 Statics
 Thermodynamics
 
©Kurt Gramoll
MATHEMATICS - CASE STUDY SOLUTION


A Circular Fin Protruding from a
Hot Wall


Point of Interest: r/ro = 0.25
and z/L = 0.5

  

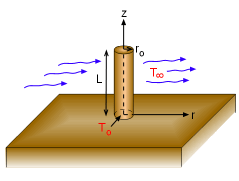
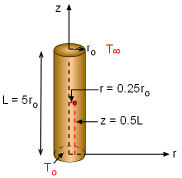
It is given that the two-dimensional steady-state temperature distribution of a circular fin is:

 

For r/ro = 0.25, L/ro = 5, and z/L = 0.5, the above equation reduces to

     
     

where the first four zeros of Jo(αn) are given as:
α1 = 2.405, α2 = 5.520, α3 = 8.654, and α4 = 11.792

The next step is to determine the Bessel functions using five terms of the power series expansion:

     

     

For α1 = 2.405, α2 = 5.520, α3 = 8.654, and α 4 = 11.792, the Bessel functions and hyperbolic sine functions are summarized in the following table:

n αn Jo(αn/4) J1(αn) sinh(2.5αn) sinh(5αn)
1 2.405 0.9116 0.5192 204.3 8.34×104
2 5.520 0.5777 0.3487 4.92×105 4.85×1011
3 8.654 0.1309 77.63 1.24×109 3.10×1018
4 11.792 -0.2388 1,767 3.18×1012 2.02×1025
     
   

The dimensionless temperature at r/ro = 0.25, L/ro = 5, and z/L = 0.5 becomes

     

Is the approximation for the Bessel functions good enough by only including five terms of the power series expansion? Use the simulation in this section to check the accuracy. Suppose that the first four zeros of Jo(α) are not given in the assumption, at least how many terms in the power series expansion do you need in order to obtain a reasonable solution?