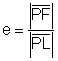| Ch 11. Polar Coordinates | Multimedia Engineering Math | ||||||
|
Polar Coordinates |
Area and Length |
Conic Sections |
|||||
| Conic Sections in Polar Coordinates | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
The parabolas, ellipses and hyperbolas will
be introduced in this section followed by the discussion of the conic
sections in polar coordinates. |
||
|
Parabolas |
|
|
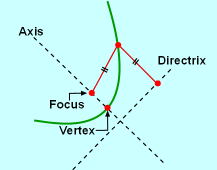
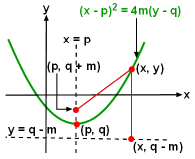
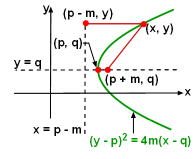
A parabola is defined as the set of points in a plane that are equidistant from a fixed point (focus) and a fixed line (directrix). The line through the focus and perpendicular to the directrix is referred to as the axis of the parabola. The point on the axis that is equidistant from the directrix and the focus is referred to as the vertex of the parabola. The vertex is also the intersection point of the parabola and the axis. The standard form of the equation for a parabola with vertex (p, q), axis x = p, directrix y = q - m and focus (p, q + m) is given by (x - p)2 = 4m(y - q) The parabola opens upward if p is positive while it opens downward when m is negative. Similarly, by interchanging the parameter x and y the parabola will be rotated 90 degrees, giving (y - p)2 = 4m(x - q) This parabola has a vertex (p, q), axis y = p, directrix x = q - m and
focus (p, q + m), as shown in the figure. The parabola opens to the right
if m is positive and to the left when p is negative. |
|
| Ellipses |
||
|
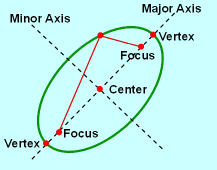
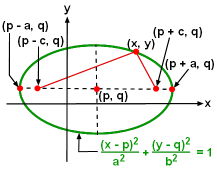
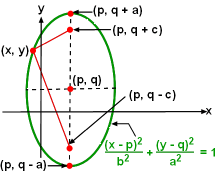
An ellipse is defined as the set of points in a plane such that the sum of the distances between a point on the ellipse and two fixed points (foci) is constant. The center of the ellipse is the midpoint of the line segment between the two foci. The points of intersection of the ellipse with the line through the foci are referred to as the vertices. The line segment between the vertices is the major axis. The minor axis is the line segment through the center and perpendicular to the major axis. If the foci of an ellipse are on the x-axis, the standard form of the equation for the ellipse is given by with center (p, q), vertices (p - a, q) and (p + a, q), foci (p - c, q) and (p + c, q). Note that a > c > 0, a > b > 0 and c2 = a2 - b2. When the foci of an ellipse are on the y-axis, the equation is given by: with center (p, q), vertices (p, q - a) and (p, q + a), foci (p, q - c) and (p, q + c). Note that a > c > 0, a > b > 0 and c2 = a2 - b2. It is noted from the above two equations that when the foci coincide, c = 0 and a = b. The ellipse becomes a circle with radius r = a = b. The eccentricity of an ellipse is defined as where 0 < e < 1. The ellipse is long and thin when e approaches 1 and the ellipse is a circle when e is close to 0. |
|
| Hyperbolas |
||
|
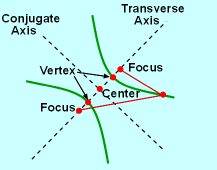
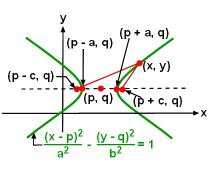
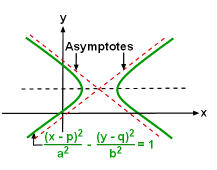
A hyperbola is defined as the set of points in a plane such that the distance difference between a point on the hyperbola and two fixed points (foci) is constant. The center of the hyperbola is the midpoint of the line segment between the two foci. The line segment through the foci is the transverse axis. The conjugate axis is the line segment through the center and perpendicular to the transverse axis. The points of intersection of the hyperbola with the transverse axis are referred to as the vertices. The hyperbola has two separate parts called the branches. The standard form of equation for a hyperbola with horizontal transverse axis is given by with center (p, q), vertices (p - a, q) and (p + a, q), foci (p - c, q) and (p + c, q). Note that c > a > 0, c > b > 0 and c2 = a2 + b2. A hyperbola will approach, but never reach, two lines called asymptotes. These lines are For a hyperbola with a vertical tranverse axis, the equation is given by: with center (p, q), vertices (p, q - a) and (p, q + a), foci (p, q - c) and (p, q + c). c > a > 0, c > b > 0 and c2 = a2 + b2. The asymptotes for the hyperbola is The eccentricity of a hyperbola is defined the same as that of an ellipse.
|
|
| Conic Sections in Polar Coordinates |
||
The three types of conic sections (i.e., parabola, ellipse and hyperbola) can be treated in a more unified form using a focus and directrix in polar coordinates. The analysis presented here assumes the focus is at the origin. Let F be a fixed point called the focus and L be a fixed line called the directrix in a plane. Let e be the eccentricity and all points P of a conic section satisfy the equation: Basically, the eccentricity is given by the ratio of the distance from
F to the distance from L. The conic section is The conic section in polar coordinates has the following form: where e is the eccentricity and d is the distance to the directrix. |
||