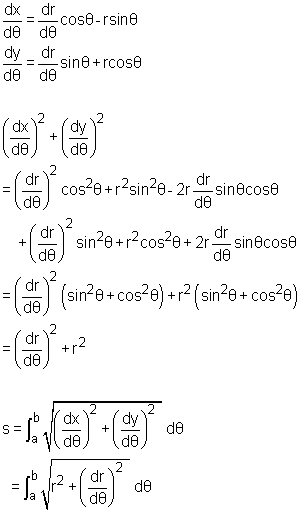| Ch 11. Polar Coordinates | Multimedia Engineering Math | ||||||
|
Polar Coordinates |
Area and Length |
Conic Sections |
|||||
| Area and Length in Polar Coordinates | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
In the previous sections, area and arc
length based on the rectangular coordinates or in parametric form
has been presented. The area and arc length in polar coordinates will
be introduced in this section. |
||
|
Area in Polar Coordinates |
|
When a curve is expressed in polar coordinates, r = f(θ), the area bounded by θ = a and θ = b is given by As an example, the area for one leaf of the four-leaved rose can be represented by r = cos(2θ) and bounded by θ = 3π/4 and θ = 5π/4. |
||
| Arc Length in Polar Coordinates |
||
|
|
When a curve is given in terms of polar coordinates r = f(θ) for a ≤ θ ≤ b, the equation can be rewritten in the parametric form as follows: x = rcosθ = f(θ)cosθ and y = rsinθ = f(θ)sinθ where θ is the parameter. According to the definition of an arc length introduced previously, the arc length of a polar curve is determined as, |
|
 Cardioid |
Take the curve of a cardioid as an example. The polar curve is represented by r = 1 + cosθ. The arc length is, |
|