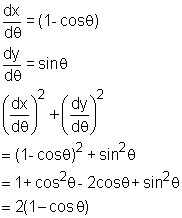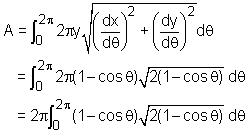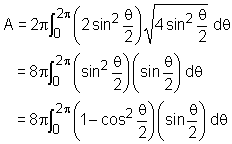Recall that when a curve is given by the following parametric equations

     x = f(t) and y = g(t) for a ≤ t ≤ b,

the surface area of revolution for the curve revolving around the x-axis is defined as

For an arch of a cycloid, the parametric equations are given by:

     x = θ - sinθ and y = 1 - cosθ
     for 0 ≤ θ ≤ 2π

where θ is the parameter.

The first step is to evaluate the derivatives appear in the square root of the equation:

The surface area is then given by

Using the trigonometry identity (half-angle formula), the above equation can be rewritten as follows:

Surface Area for an Arch of a Cycloid
Revolving Around an x-Axis.

The above integral can be evaluated through the change of variable (let t = θ/2):

From the tables of integration, the integration can be performed to yield