| Ch 9. Integrate Application | Multimedia Engineering Math | ||||||
|
Area Between Curves |
Volume | Work | Average Value | ||||
| Average Value of a Function | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
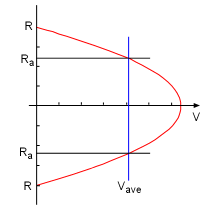
A sensor needs to be installed in a location where the velocity equals the average velocity of the whole cross-sectional area of a blood vessel. Also, the average velocity needs to be determined. |
||
 Velocity Function v(r) = [P / (4μl)] (R2 - r2) |
The velocity function v(r) = [P / (4μl)] (R2 - r2) is continuous on the interval [0, R], The mean Value Theorem for Integrals says there is a number Ra in [0, R] such that Solve for Ra from the above equation gives Ra = 0.5774R = 0.5774(0.08
x 10-3) |
|
The average velocity equals the velocity at r = Ra. vave = v(0.0462 x 10-3)
= P/(4μl)(R2 -
Rar2) The average velocity is 2.132 mm/s and the sensor can be installed at 0.0462 mm from the center. |
||