The boundary layer theory will be introduced in this section through a discussion of the characteristics of flow past a flat plate.

Characteristics of Flow Past a Finite Flat Plate Subject to Different Reynolds Numbers:
(a) Re = 0.1, (b) Re = 10 and (c) Re = 10⁷

Boundary Layer Thickness

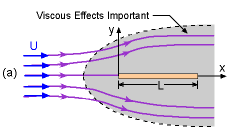
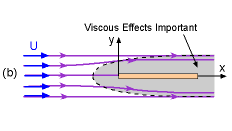
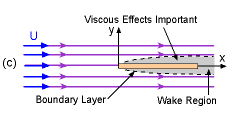
When fluid flows past an immersed body, a thin boundary layer will be developed near the solid body due to the no-slip condition (i.e., fluid is stuck to the solid boundary). The flow can be treated as inviscid flow outside of this boundary layer, while viscous effects are important inside of this boundary layer.

Take flow past a flat plate for example. The characteristics of flow past a flat plate with finite length L subject to different Reynolds numbers (Re = ρUL/μ) are shown in the figures. At a low Reynolds number (Re = 0.1), the presence of the flat plate is felt in a relatively large area where the viscous effects are important .

At a moderate Reynolds number (Re = 10), the viscous layer region becomes smaller. Viscous effects are only important inside of this region, and streamlines are deflected as fluid enters it.

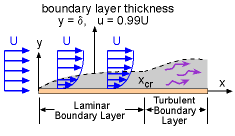
As the Reynolds number is increased further (Re = 10⁷), only a thin boundary layer develops near the flat plate, and the fluid forms a narrow wake region behind the flat plate. Hence, the flow can be considered as inviscid flow everywhere except the boundary layer region.

Now consider flow past an infinite long flat plate with neglegible thickness. In this case, the Reynolds number is defined using the local distance x (i.e., the distance from the leading edge along the flat plate as the characteristic length). The local Reynolds number is then given by

     Re_x = ρUx/μ

As the fluid flows past the long flat plate, the flow will become turbulent at a critical distance x_cr downstream from the leading edge. For flow past a flat plate, the transition from laminar to turbulent begins when the critical Reynolds number (Re_xcr) reaches 5×10⁵. The boundary layer changes from laminar to turbulent at this point.

The concept of a boundary layer was introduced and formulated by Prandtl for steady, two-dimensional laminar flow past a flat plate using the Navier-Stokes equations. Prandtl's student, Blasius, was able to solve these equations analytically for large Reynolds number flows. The details of the derivation are omitted for simplicity, and the results are summarized here.

Based on Blasius' analytical solutions, the boundary layer thickness (δ) for the laminar region is given by

     

where δ is defined as the boundary layer thickness in which the velocity is 99% of the free stream velocity (i.e., y = δ, u = 0.99U).

The wall shear stress is determined by

If this shear stress is integrated over the surface of the plate ares, the drag coefficient for laminar flow can be obtained for the flat plate with finite length as

If the flow is turbulent, then the equations for boundary layer and drag coefficient is