| Ch 9. Integrate Application | Multimedia Engineering Math | ||||||
|
Area Between Curves |
Volume | Work | Average Value | ||||
| Volume | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
| Introduction |
||
|
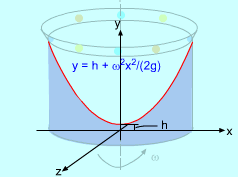
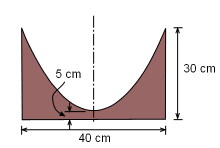
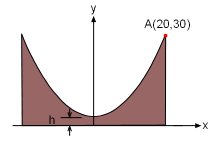
In his pottery class, Roy noticed that when cylindrical container with clay is rotated, the clay forms a parabolic shape. After some experimenting, he notices that the surface of the clay is a paraboloid of revolution generated by rotating the parabola y = h + ω2 x2/(2g) about the y-axis, where ω is the angular velocity and g is the gravitational acceleration. He now wonders how he can determine the angular velocity so that the clay will conform to a given height and width. He would like to use this for a mold. What is known: Mold size required is 30 cm high and 40 cm wide as shown in the diagram at the left. |
|
|
Questions |
|
What is the angular velocity required so the clay is 30 cm high at the outside edge and 5 cm high at the center? Also, what is the total volume of clay needed? |
||
|
|
Approach |
|
|
||