| Ch 9. Friction | Multimedia Engineering Statics | ||||||
| Friction I | Friction II | ||||||
| Friction I | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
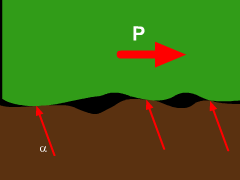
 Friction on a Block in Equilibrium |
In previous sections, it was assumed that objects were frictionless. Consequently, the only reaction forces were normal to the surface. This ideal approximation always involves relative errors because no surface is perfectly frictionless. There are several types of frictional forces used in mechanics. However, dry friction will be the primary form considered in this section.
|
|
| Dry Friction |
||
|
|
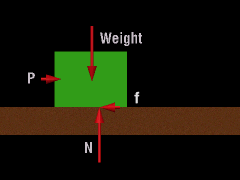
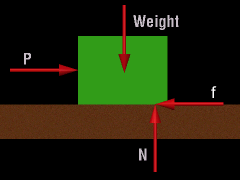
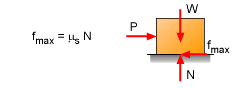
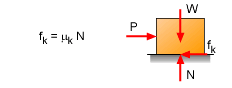
Dry friction, also known as Coulomb friction, occurs between two rough, solid surfaces. Frictional forces always oppose the tendency towards motion and act tangential to the surfaces in contact. Static friction describes surfaces that are in contact but not moving. The maximum static friction is represented by fmax = μs N Here, μs is a proportionality constant called the coefficient of static friction. This equation only gives the force of friction when motion is impending. If motion is not impending, then the friction force, fs, will be less than the maximum, or fs ≤ μs N Thus, only when motion is just starting to take place will the friction force be a maximum. On the other hand, if motion has started, then kinetic friction is used, or fk = μk N Here, μk is the coefficient of kinetic friction. This equation only applies when an object is in motion. It is generally true that μk is less than μs and may vary slightly with velocity. In general, friction simply written as f = μ N It is also helpful to consider the resultant of the frictional and normal forces. The angle α from the normal force to the resultant can be calculated as tanα = f/N = μ As the static friction approaches fmax, α reaches a maximum angle φs. tanφs = μs Likewise, a similar equation for kinetic friction can be written as tanφk = fk/N = μk The angles φs and φk define the limiting positions of the reactions between surfaces. There are typically three types of friction problems. |
|
 |
Type 1: Impending Motion |
|
|
Impending motion is known to exist because the system is on the verge of slipping. The equilibrium equations and basic equation Fmax = μs N hold true. |
||
| Type 2: Status Unknown |
||
 |
Neither impending motion nor motion is known. Using equilibrium equations, the friction force direction on a surface is assumed and solved. Comparing the results give
|
|
 |
Type 3: Relative Motion |
|
|
Relative motion exists between the surfaces. Therefore, the properties of kinetic friction would be in effect. |
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.