| Ch 9. External Flow | Multimedia Engineering Fluids | ||||||
| Drag | Lift |
Boundary Layer |
|||||
| Drag | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Fluid Statics |
| 3. Kinematics |
| 4. Laws (Integral) |
| 5. Laws (Diff.) |
| 6. Modeling/Similitude |
| 7. Inviscid |
| 8. Viscous |
| 9. External Flow |
| 10. Open-Channel |
| Appendix |
| Basic Math |
| Units |
| Basic Fluid Eqs |
| Water/Air Tables |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||||||||||||||||||||||||||||||||||||||||||||||||
|
In this section, the concept of drag will be introduced, and the concept of flow over an immersed body is illustrated with flow over a sphere. |
||||||||||||||||||||||||||||||||||||||||||||||||
|
|
Drag |
|||||||||||||||||||||||||||||||||||||||||||||||
|
When fluid flows over an immersed body,
forces are exerted on that body. The resultant force parallel to the fluid motion is referred to
as the drag. Determining drag is important in many engineering applications,
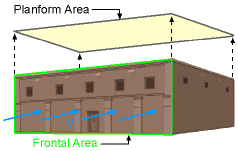
such as the design of automobiles, airplanes, submarines and buildings. where ρ is the fluid density; A is the characteristic area and V is the upstream velocity. The characteristic area can be choosen as: (a) the projected frontal area (often used for flow over automobiles and submarines), (b) the planform area (often used for flow over wings and hydrofoils), and (c) the wetted area (often used for flow over ships and barges). Hence, it is important to know which characteristic area was used when determining the drag coefficient. Generally, the drag coefficient is determined through experiments, and it depends on parameters, such as the body shape, Reynolds number and surface roughness. The value of the drag coefficient for different body shapes subject to different flow conditions are available in the literature. Some of the drag coefficients for flow over two-dimensional and three-dimensional bodies are summarized in the following tables. |
||||||||||||||||||||||||||||||||||||||||||||||||
Drag Coefficient for
Two-Dimensional Body
|
Drag Coefficient for
Three-Dimensional Body
|
|||||||||||||||||||||||||||||||||||||||||||||||
| Flow over a Sphere |
||||||||||||||||||||||||||||||||||||||||||||||||
|
|
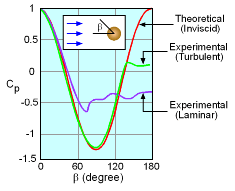
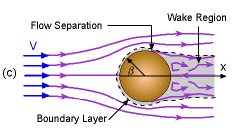
Consider flow over a sphere for illustration. Unlike
flow over a flat plate,
the pressure field of flow over a sphere is not uniform. This can be
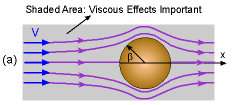
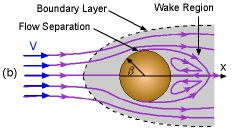
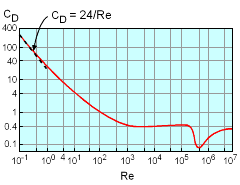
seen from the pressure distribution (i.e., At low Reynolds numbers (i.e., Re << 1), the viscous effects are important in a large area (shaded area). There is no flow separation, and the fluid is stuck to the sphere. The drag coefficient as a function of the Reynolds number is shown in the figure. At very small Reynolds numbers, Stokes has shown that the drag coefficient is a linear function of the Reynolds number, as given by CD = 24/Re At moderate Reynolds number (103 < Re < 2×105), a boundary layer is developed near the sphere. Viscous effects are important inside the region of this boundary layer. Due to the increase of the pressure drag, the fluid can no longer stick to the sphere, and it is separated at an angle (β) of about 80o. A broad wake region is formed downstreams. Note that the drag coefficient decreases with the Reynolds number, and it becomes almost a constant (CD = 0.4) for a Reynolds number between 103 and 2×105. As the Reynolds number increases (Re > 2×105), the boundary layer becomes thinner in the front of the sphere and begins its transition to turbulent. The flow separation is delayed until an angle of about 120o, and the fluid forms a relatively narrow wake region in which the flow is highly unsteady and turbulent. For turbulent boundary layer flow, the drag coefficient is decreased further (e.g., CD = 0.06 for Re = 4×105). Hence, a turbulent boundary layer develops as fluid flows past an object will reduce the drag force. For example, the dimples of a golf ball (i.e., the surface roughness of the object) are used to create turbulent boundary layer flow, and hence reduce the drag force.
|
|||||||||||||||||||||||||||||||||||||||||||||||
| Numerical Solutions to Low Reynolds Number Flows |
||||||||||||||||||||||||||||||||||||||||||||||||
For Android mobile devices, "Flow HPC", can be downloaded at Google Play. For iOS (Apple) mobile devices, "Flow HPC", can be downloaded at iTunes. |
Determining drag for unique shapes can be relatively difficult even for numerical methods. Basically, the full nonlinear Navier-Stokes equations must be solved. For low Reynolds number it is manageable using finite element method. A simple demonstration simulation is given below. It currently only works for Re < 50. | |||||||||||||||||||||||||||||||||||||||||||||||
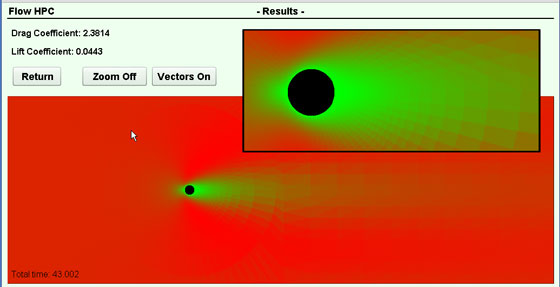 Example of the FlowHPC Drag Simulation Mobile App |
||||||||||||||||||||||||||||||||||||||||||||||||
Practice Homework and Test problems now available in the 'Eng Fluids' mobile app
Includes over 250 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.