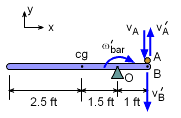
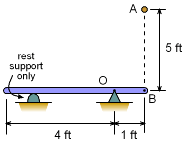
A slender bar, B, has a mass of 2 slugs and is pinned at O. A 2 slug particle, A, is dropped from a height of 5 ft and strikes the bar at the far right end. What is the angular velocity of the bar just after impact? Assume the coefficient of restitution, e, is 1.

Velocity Diagram

First, find the velocity of the particle just before it strikes the bar. From conservation of energy,
    T₁ + V₁ = T₂ + V₂
     0.5mv_A² + 0 = 0 + mgh
     v_A = [32.2(5) / 0.5]^0.5 = 17.94 ft/s (down)

The impact will transfer the motion of the particle to the motion of the bar attached,
     e = [(-v'_B) - v'_A] / [(-v_A) - v_B]
     1 = (-v'_B - v'_A) / [-17.94 - 0]
     17.94 = v'_B + v'_A

However, the linear velocity of B is related to the angular velocity of the bar,
      -v'_B = - ω'_B r_OB
      v'_B = ω'_B (1 ft)

Combining gives,
      17.94 = ω'_B + v'_A                             (1)

The angular momentum of the bar (with the particle attached) must be conserved, giving,
     ΣH_o = ΣH'_o
     m_A (-v_A) r = m_A v'_A r + I_o (-ω'_B)
     2 (-17.94)(1) = 2 (v'_A)(1) + I_o(-ω'_B)
     -35.89 = 2v'_A - I_oω'_B                           (2)

Mass moment of inertia, I_o
     I_o = mL²/12 + m(1.5)² = 2(5)²/12 + 2(2.25)
     I_o = 8.667 slug-ft²                              (3)

Combining with Eqs. 1, 2 and 3 gives,
     17.94 = ω'_B + (-35.89 + 8.667ω'_B) / 2

ω'_B = 6.729 rad/s