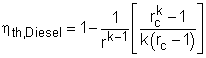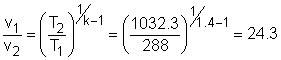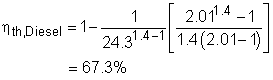| Ch 8. Gas Power Cycle | Multimedia Engineering Thermodynamics | ||||||
|
Otto Cycle |
Diesel Cycle |
||||||
| Diesel Cycle | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Pure Substances |
| 3. First Law |
| 4. Energy Analysis |
| 5. Second Law |
| 6. Entropy |
| 7. Exergy Analysis |
| 8. Gas Power Cyc |
| 9. Brayton Cycle |
| 10. Rankine Cycle |
| Appendix |
| Basic Math |
| Units |
| Thermo Tables |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
In Max's senior capstone design, the thermal efficiencies and the compression ratios of an ideal Otto cycle and an ideal Diesel cycle are required. Assumptions:
|
||
|
|
(1) Determine the thermal efficiency and compression ratio using ideal Otto-cycle model The P-v and T-s diagrams of the ideal Otto cycle are shown on the left. The thermal efficiency and compression ratio using the Otto-cycle model has been determined in the previous section. They are: Thermal efficiency: ηth, Otto = 53.3% Compression ratio: r = 6.7 |
|
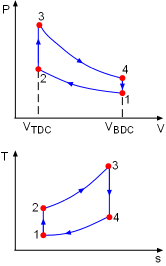
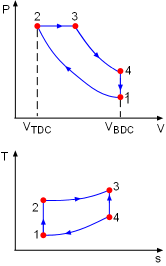 P-v and T-s Diagram of the Diesel Cycle |
(2) Determine the thermal efficiency and compression ratio using Diesel-cycle model The P-v and T-s diagrams of the ideal Diesel cycle are shown on the left. The previous section, the properties at the four states of an Otto cycle was determined. They are: state 1: T1 = 15oC,
P1 = 100 kPa (given) The heat input to the cycle is: qin,Otto = cv23 (T3 -
T2) = 0.718(1800 - 343.3) In Diesel cycle, with the temperature limit is the same as In Otto cycle, temperature at state 1 and state 3 are: T1 = 15oC Also, heat input is the same as in the ideal Otto cycle. In Diesel cycle, heat is input from the constant pressure cycle. qin,Diesel = cP23 (T3 -
T2) = 1.005 (1800 - T2) The temperature at state 2 can be determined from the above expression. That is, T2 = 759.3oC = 1032.3 K The thermal efficiency of the ideal Diesel cycle is: where r is the compression ratio and rc is the cutoff ratio. r = v1/v2 In Diesel cycle, process 1-2 is isentropic compression process. It gives, |
|
Hence, the compression ratio of an ideal Diesel-cycle is 24.3, which is much higher than the compression ratio of an ideal Otto-cycle, which is 6.7. Process 2-3 in an ideal Diesel cycle is an constant pressure cycle. Thus, It gives that the cutoff ratio equals 2.01. Substitute the compression ratio and cutoff ratio to the expression of thermal efficiency yields, Also, the thermal efficiency of the ideal Diesel-cycle is much higher than the ideal Otto-cycle, which is 53.3%. |
||