| Ch 8. Rigid Body Momentum/Impulse | Multimedia Engineering Dynamics | ||||||
| Impulse Momentum | Eccentric Impact | ||||||
| Principle of Impulse and Momentum | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
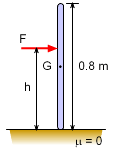 Thin Vertical Rod |
Example |
|
|
A thin rod having a mass of 4 kg is balanced vertically as shown. Determine the height h at which it can be struck with a horizontal force F so that it rotates but does not slip on the floor. Assume the coefficient of friction is zero. |
||
| Solution |
||
|
|
The horizontal linear impulse and momentum is, The rod will not slip, so it will rotate about its bottom point where it touches the ground, Since the rod will also rotate, the angular impulse and momentum equation must also apply about its center of mass. The term, "F Δt" can be eliminated by combining the two equations, giving, Solving for h gives, h = 0.5333 m |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.