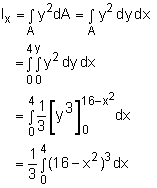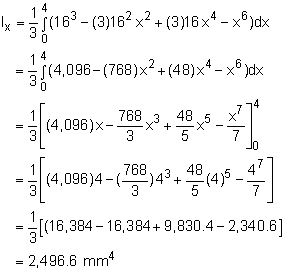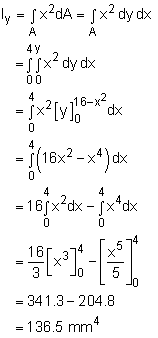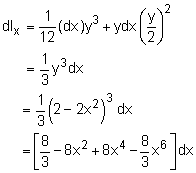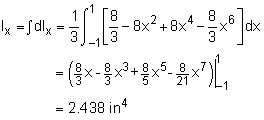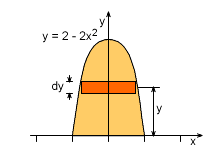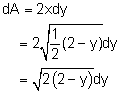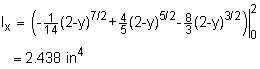| Ch 7. Centroid/Distributed Loads/Inertia | Multimedia Engineering Statics | ||||||
|
Centroid: Line Area Vol |
Centroid: Composite | Distributed Loads | Area Moment of Inertia | ||||
| Area Moment of Inertia | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| STATICS - EXAMPLE |
||
|
|
Example 1 |
|
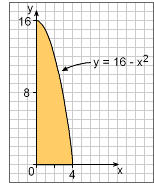
Find moment of inertia of the shaded area about
|
||
| Solution (a) |
||
Recall, the moment of inertia is the second moment of the area about a given axis or line. For part a) of this problem, the moment of inertia is about the x-axis. The differential element, dA, is usually broken into two parts, dx and dy (dA = dx dy), which makes integration easier. This also requires the integral be split into integration along the x direction (dx) and along the y direction (dy). The order of integration, dx or dy, is optional, but usually there is an easy way, and a more difficult way. |
||
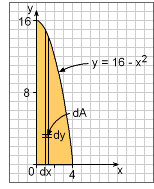 Cross-section Area |
For this problem, the integration will be done first along the y direction, and then along the x direction. This order is easier since the curve function is given as y is equal to a function of x. The diagram at the left shows the dy going from 0 to the curve, or just y. Thus the limits of integration is 0 to y. The next integration along the x direction goes from 0 to 4. The final integration from is Expanding the bracket by using the formula, | |
| Solution (b) |
||
Similar to the previous solution is part a), the moment of inertia is the second moment of the area about a given axis or line. But in this case, it is about the y-axis, or | ||
 Cross-section Area |
The integral is still split into integration along the x direction (dx) and along the y direction (dy). Again, the integration will be done first along the y direction, and then along the x direction. The diagram at the left shows the dy going from 0 to the curve, or just y. Thus the limits of integration is 0 to y. The next integration along the x direction goes from 0 to 4. The final integration from is | |
| Comment | ||
| The area is more closely distributed about the y-axis than x-axis. Thus, the moment of inertia of the shaded region is less about the y-axis as compared to x-axis. | ||
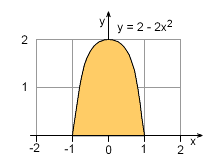 |
Example 2 |
|
Determine the moment of inertia of y = 2 - 2x2 about the x axis. Calculate the moment of inertia in two different ways. First, (a) by taking a differential element, having a thickness dx and second, (b) by using a horizontal element with a thickness, dy. |
||
| Solution |
||
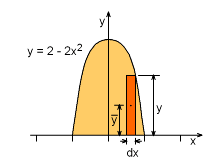 |
a) The area of the differential element parallel to y axis is dA = ydx. The distance from x axis to the center of the element is namedy. y = y/2 Using the parallel axis theorem, the moment of inertia of this element about x axis is For a rectangular shape, I is bh3/12. Substituting Ix, dA, and y gives, Performing the integration, gives, |
|
|
(b) First, the function should be rewritten in terms of y as the independent variable. Due to the x2 term, there is a positive and negative form and it can be expressed as two similar functions mirrored about y axis. The function on the right side of the axis can be expressed as The area of the differential element parallel to x axis is Performing the integration gives, Performing a numerical integration on calculator or by taking t = 2(2 - y) the above integration can be found as, As expected, both methods (a) and (b) provide the same answer. |
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.