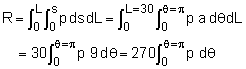| Ch 7. Centroid/Distributed Loads/Inertia | Multimedia Engineering Statics | ||||||
|
Centroid: Line Area Vol |
Centroid: Composite | Distributed Loads | Area Moment of Inertia | ||||
| Distributed Loads | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Pressure Distribution |
||
|
|
The pressure at a point on the submerged arch is perpendicular to the surface and depends on the water depth at that point. If an axis system is oriented at the center of the arch, the pressure at any height y can be determined as p = ρg(b - y) where ρ is the water density, g is the acceleration of gravity and b is the total depth of the water to the floor. If the angle θ is as shown, then y = a sinθ and p = ρg(b - a sinθ) The pressure at any angle θ can be broken into x and y components as px = -p cosθ py = -p sinθ |
|
| Resultant Force |
||
 Integrate Over Surface Area S |
The resultant force is found by integrating the pressure over the arch surface, S, which means integrating θ from 0 to π and multiplying by the length of the arch. For the x component:
Integrate or use integral tables to find Rx = 270 ρg [a/2 sin2θ - b sinθ]0π Evaluating the integral for Rx gives Rx = 0 lb The y component is
Integrating and evaluating for Ry Ry = 270ρg [a/2 (-cosθ sinθ + θ) b cosθ]0π Ry = 270ρg (aπ/2 - 2b) lb Finally, substitute for the known density, water depth, and arch radius to give Ry = -436,200 lb = -436 kips Therefore, in vector form, the resultant force is R = 0i - 436j + 0k kips |
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.