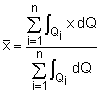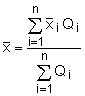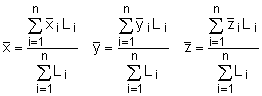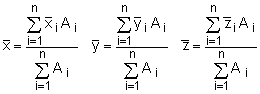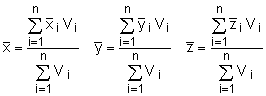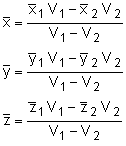| Ch 7. Centroid/Distributed Loads/Inertia | Multimedia Engineering Statics | ||||||
|
Centroid: Line Area Vol |
Centroid: Composite | Distributed Loads | Area Moment of Inertia | ||||
| Centroid: Composite Parts | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Equilibrium |
||
|
In the previous section, Centroid: Line, Area and Volume , it was shown that the centroid of an object involves evaluating integrals of the form where Q is a line, area, or volume, depending on the centroid that is required. The same equation can also be used for the other two directions by just substituting y or z for x. If there are several objects, then the centroid of the entire system is given by where n is the number of objects in the system. This equation can be simplify as where |
||
| Centroid of a System of Lines |
||
|
For a system of lines, the coordinates of the centroid are Here |
||
| Area Centroid of a System of Objects |
||
|
For a system of objects, the coordinates of the area centroid are Here |
||
| Volume Centroid of a System of Objects |
||
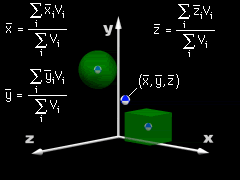 Centroid of Multiple Objects |
For a system of objects, the coordinates of the volume centroid are Here |
|
| Subtraction of Material (Holes) |
||
|
If an object or system of objects has a cutout or hole, then the centroid of the system can be found by considering the cutout or hole as a negative area, volume, or line length. For example, if a system consists of a cube with a centroid at x1, y1, z1 and a volume of V1; and a hole within the cube that has a centroid at x2, y2, z2 and a volume of V2; then the coordinates of the centroid for the system are |
||
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.