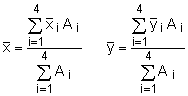| Ch 7. Centroid/Distributed Loads/Inertia | Multimedia Engineering Statics | ||||||
|
Centroid: Line Area Vol |
Centroid: Composite | Distributed Loads | Area Moment of Inertia | ||||
| Centroid: Composite Parts | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
|
|
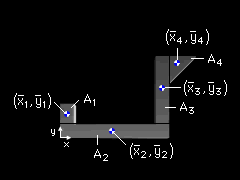
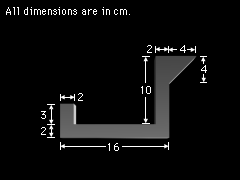
The beam cross section can be broken into 4 composite parts consisting of 3 rectangles and a triangle as shown. Orient an axis system as shown, so that the cross section lies entirely in the x-y plane. Since the thickness is constant for all the parts, the centroid can be found using the area equations,
|
|
| Composite Part 1 |
||
|
|
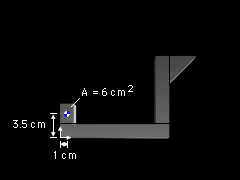
Part 1 is a rectangle with an area of A1 = (2)(3) = 6 cm2 The centroid of a rectangle lies at half its width and half its height, so for part 1
|
|
| Composite Part 2 |
||
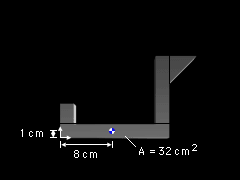 Part 2 |
Part 2 is a rectangle with an area of A2 = (16)(2) = 32 cm2 Its centroid is located at
|
|
| Composite Part 3 |
||
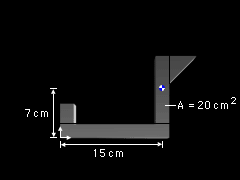 Part 3 |
Part 3 is a rectangle with an area of A3 = (2)(10) = 20 cm2 Its centroid is located at
|
|
| Composite Part 4 |
||
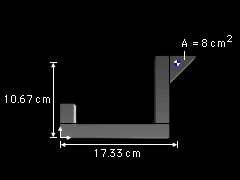 Part 4 |
Part 4 is a triangle with an area of A4 = (0.5)(4)(4) = 8 cm2 The centroid of a right triangle is located two-thirds of the distance from the vertex to the other end; therefore, for part 4
|
|
| Centroid of Total System |
||
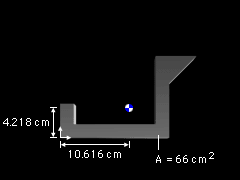 Total System |
With these results, find the total area of the system is Substituting the areas and centroid locations for each of the individual parts into the first two equations gives
|
|
Practice Homework and Test problems now available in the 'Eng Statics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.