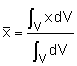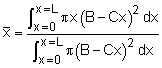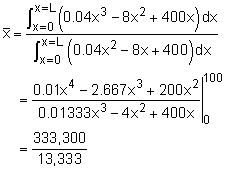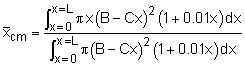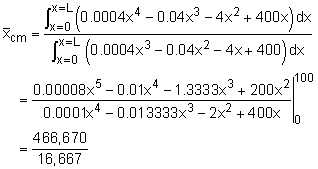| Ch 7. Centroid/Distributed Loads/Inertia | Multimedia Engineering Statics | ||||||
|
Centroid: Line Area Vol |
Centroid: Composite | Distributed Loads | Area Moment of Inertia | ||||
| Centroid: Line, Area and Volume | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Centroid |
||
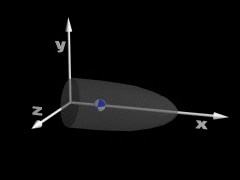 Centroid |
For the first approximation, the radius of the nose cone is given by the equation (recall, D is assumed to be 1) r(x) = B - Cx ft Since the radius is known to be 20 ft at x = 0, the constant B can be determined as r(0) = B - C(0) => B = 20 At the other end, the nose cone tapers to zero, so at x = 100 ft, the radius is zero. Using this condition, the constant C can be determined, r(100) = 20 - 100 C = 0 C = 0.2 Based on symmetry, the centroid must lie somewhere along the x axis. |
|
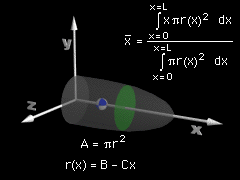 Integrating the Volume |
The x coordinate of the centroid using the equation, To perform the integrations, a slice of the nose cone that is perpendicular to the x axis can be used. For any slice, the area is A(x) = π r(x)2 The volume will be the area times the slice thickness, dx, giving V(x) = π r(x)2 dx Combining equations gives Here L is the length of the nose cone. Expand the numerator and denominator and integrate gives The final result is |
|
| Center of Mass |
||
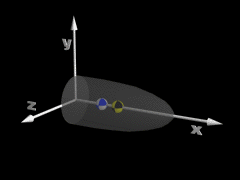 Centroid and Center of Mass |
Since the density varies from 1 slug/ft3 at the base to 2 slug/ft3 at the tip, the density is given by the equation ρ(x) = 1 + 0.01x slug/ft3 The density does not vary in the y or z direction, therefore Substitute the density and area equations into the center of mass equation gives Expand and integrate gives
The final result is Notice the distance is slightly larger than the centroid, which corresponds to the increasing density toward the front. |
|
| Center of Gravity |
||
|
Since the nose cone is relatively small, variation of gravity over its volume can be neglected. Thus, the center of gravity and the center of mass are the same point.
|
||