| Ch 6. Entropy | Multimedia Engineering Thermodynamics | ||||||
| Entropy | Tds Relations |
Entropy Change |
Isentropic Process |
Isentropic Efficiency |
Entropy Balance (1) |
Entropy Balance (2) |
Reversible Work |
| Isentropic Efficiency | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Basics |
| 2. Pure Substances |
| 3. First Law |
| 4. Energy Analysis |
| 5. Second Law |
| 6. Entropy |
| 7. Exergy Analysis |
| 8. Gas Power Cyc |
| 9. Brayton Cycle |
| 10. Rankine Cycle |
| Appendix |
| Basic Math |
| Units |
| Thermo Tables |
| Search |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| Author(s): |
| Meirong Huang |
| Kurt Gramoll |
| ©Kurt Gramoll |
|
|
||
|
|
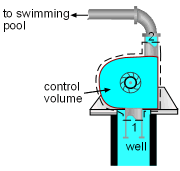
A used pump must match the motor in Mr. Williams' swimming pool. The power required to run the pump needs to be determined. Assumptions:
|
|
|
|
Take the pump as a control volume, shown on the left. Denote the inlet by subscript 1 and exit by subscript 2. The definition of isentropic efficiency of pump is: η P It is assumed that the pump operates at ambient temperature. Hence the specific volume of water is v = 0.001 m3/kg The pump increases the pressure of the water up to 300 kPa, which means the pressure difference of water between the exit and inlet is 300 kPa. That is P2 - P1 = 300 kPa Substituting the given efficiency, which is 85%, and the pressure difference above to the definition of isentropic efficiency gives, h2 - h1 = v(P2 - P1)/η P With the assumptions that the pump is adiabatic and the kinetic and potential energies are negligible, the energy balance of the pump reduces to The mass flow rate is given as 20 kg/min. Substituting the enthalpy difference and the mass flow rate to the energy balance yields, The negative sign means the pump needs power input. |
|