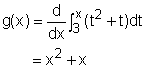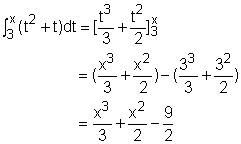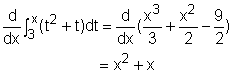| Ch 6. Integrals | Multimedia Engineering Math | ||||||
|
Indefinite Integral |
Area |
Definite Integral |
Fundamental Theorem |
Substitution Rule |
|||
| Fundamental Theorem of Calculus | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||||||||||||||||||||||
This section introduces the Fundamental Theorem of Calculus. |
||||||||||||||||||||||
| Fundamental Theorem of Calculus |
||||||||||||||||||||||
The concept of derivative and integral will be related by the Fundamental Theorem of Calculus. This theorem consists of two parts.
|
||||||||||||||||||||||
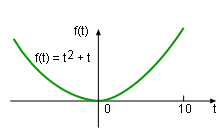 Continuous Function f(t) = t2 + t |
This theorem can be better understood by finding the
result of The function t2 + t is a continuous function over the interval between 3 and x. According to the first part of Fundamental Theorem of Calculus, the derivative of this function is |
|||||||||||||||||||||
In order to double check the answer, |
||||||||||||||||||||||
|
Next, take the derivative of the result gives It is clear that both methods give the same results. In order to use the Fundamental Theorem of Calculus effectively, important formulas of antiderivative are given in the table on the left. |
|||||||||||||||||||||