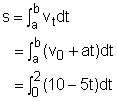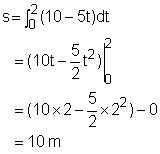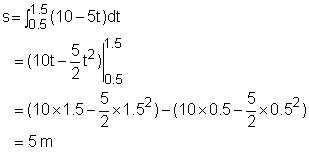| Ch 6. Integrals | Multimedia Engineering Math | ||||||
|
Indefinite Integral |
Area |
Definite Integral |
Fundamental Theorem |
Substitution Rule |
|||
| Fundamental Theorem of Calculus | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
The car has an initial speed of 10m/s when the traffic light turns red and starts to decelerate at -5 m/s2. How far does the car travel before it stops? Let the velocity, distance and time to be v, s and t respectively. The
velocity at 0 and t second is represented by v0 and vt respectively. |
||
In order to find the traveling distance, the time spent on stopping the car should to be calculated first. The velocity function is vt = v0 + at in which v0 = 10, vt = 0 and a = -5. Substituting v0, v0 and a into the above equation gives t = 2.0 The stopping distance is the integral of velocity with respect to time, that is In the above equation, the lower limit is the time that the car starts
to brake, which is 0 seconds. The upper limit is the time that the car
stops, which is 2 seconds.The Fundamental Theorem of Calculus gives The distance the car travels can be calculated |
||
| Discussion |
||
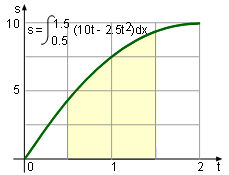 Braking Distance in [0.5, 1.5] |
The braking distance for different intervals can be calculated by changing the upper and lower limit of the integral. For example, the braking distance between 0.5 to 1.5 seconds in the above case is |
|