| Ch 6. Rigid Body Force and Acceleration | Multimedia Engineering Dynamics | ||||||
|
Moment of Inertia |
Translational Motion | Fixed Point Rotation |
General Plane Motion |
||||
| General Plane Motion | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Solution of a) |
||
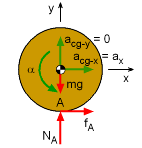 Problem Diagram
|
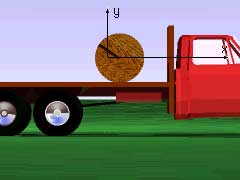
As with all dynamics problems, first start with a detailed diagram showing all forces and accelerations involved ( shown at the left). Summing the forces acting on the spool give ΣFx => fA = max ΣFy => NA - mg = 0 Since the spool rotates about its center of gravity, the moments are summed at the center, giving ΣMcg => fAr = Icg α There are four unknowns (fA, ax, NA, α) but only three equations. Thus, to complete the solution, a fourth equation must be derived that relates the acceleration of the spool's center of gravity to the angular acceleration. Because the spool does not slip, the acceleration of point A can be related to the acceleration of the cg point, acg = aA + acg/A |
|
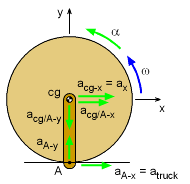 Acceleration Vectors for Link Between Points cg and A |
acg-xi + 0j = [aA-xi + aA-y j] + [acg-xi - acg-y j] axi + 0j = [atrucki + ω2r j] + [α r i - ω2r j]
ax = atruck - rα There are now four equations to solve for the four unknowns (fA, ax, NA, α). Combining gives two equations, (max) r = Icgα Reducing to one equation, mr (atruck - rα) = Icgα or α = m r atruck / [Icg + mr2] and ax = atruck - rα = 1 - 0.5(1.333) = 0.3333 m/s Thus, the spool accelerates to in the same direction as the truck acceleration even though it rolls backwards with an angular acceleration of 1.333 rad/s2. |
|
| Solution of b) |
||
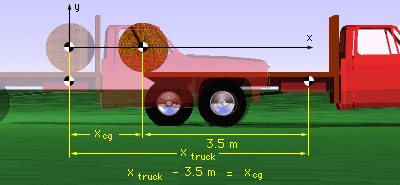 Position Diagram |
||
|
|
To determine the time it takes for the spool to reach the end of the truck, it helps to draw a diagram of the spool at the end of the truck. From the diagram, when the spool reaches the end of the truck, the following relationship is true, xtruck - 3.5 m = xcg Use the acceleration of the center of gravity of the spool and the acceleration of the truck to integrate to find xcg and xtruck, xcg(t) = 0.167 t2 xtruck(t) = 1/2 t2 Substituting the expressions for xcg and xtruck, gives 1/2 t2 - 3.5 = 0.167 t2 Solving for t, t = 3.24 s |
|
Practice Homework and Test problems now available in the 'Eng Dynamics' mobile app
Includes over 400 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.