| Ch 6. Integrals | Multimedia Engineering Math | ||||||
|
Indefinite Integral |
Area |
Definite Integral |
Fundamental Theorem |
Substitution Rule |
|||
| Definite Integral | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
This section introduces the definition of the integral and its properties. |
||
| Definite Integral |
||
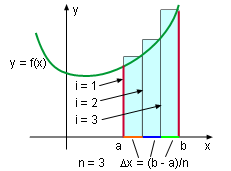
| In Area section, the limit was used to approximate the area between a curve and x-axis. This type of limit often occurs in engineering research, such as finding the fluid pressure over an area or the work used in a process. A special name and notation is given to this kind of limit - definite integral. It states: | ||
 Definite Integral |
If a function f is defined on a closed interval between a and b, and it is equally divided into n parts. So the length of each part is Δx = (b-a)/n, then the definite integral of function f from a to b is In this equation, ∫ is the integration sign; a is the lower limit;
b is the upper limit; f(x) is the integrand. The procedure
for calculating an integral is called integration and |
|
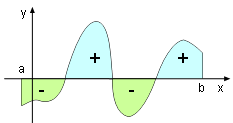
The definite integral of the function f(x), = the area under the graph of function from a to b |
||
 Integral and area 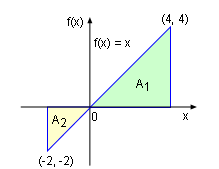 Integral and area |
In general, a definite integral can be interpreted as the difference of areas: in which A1 is the sum area of the region above the x-axis and A2 is the sum area of the region below the x-axis as shown on the left. For example, the integral function f(x) = x between -2 and 4 can be calculated as the difference of the the two triangles. Although this problem can be calculated, some of the integral can't be calculated. |
|
| Integrable |
||
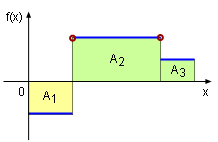 Integrable Discontinuous Function |
It is clear that, if Any function that is integrable between a and b can be expressed as |
|
| Midpoint Rule |
||
In order to calculate the limit, the right endpoints are generally used. If the midpoint is used, the limit will be an approximate value and the integral will be where Δx = (b - a)/n and To assist in evaluating integrals, some basic properties of integrals are list below. |
||
| Properties of the Integral |
||
| If the integral of a function exist in [a, b], then | ||
|
||