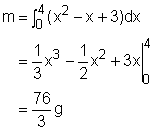| Ch 6. Integrals | Multimedia Engineering Math | ||||||
|
Indefinite Integral |
Area |
Definite Integral |
Fundamental Theorem |
Substitution Rule |
|||
| Definite Integral | Case Intro | Theory | Case Solution |
| Chapter |
| 1. Limits |
| 2. Derivatives I |
| 3. Derivatives II |
| 4. Mean Value |
| 5. Curve Sketching |
| 6. Integrals |
| 7. Inverse Functions |
| 8. Integration Tech. |
| 9. Integrate App. |
| 10. Parametric Eqs. |
| 11. Polar Coord. |
| 12. Series |
| Appendix |
| Basic Math |
| Units |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
| |
||
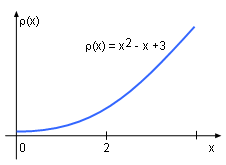 The Linear Density Function of the rod |
The linear density of a non-homogeneous rod is expressed as ρ(x) = x2 - x + 3 with unit of g/m. If the rod length is 4 m, what is the mass of the rod? If the rod is divided into many small pieces, the total mass of the rod is the sum of the mass in each piece, which can be expressed as When the rod is divided into infinite small pieces, the result is the exact mass value. |
|
 The Low and Upper Limit of rod |
The definite integral definition gives Therefore, in which x1 and x2 are the start and the end of the rod. Substituting x1 = 0, x2 = 4, and ρ(x) = x2 - x + 3 into the above formula gives |
|