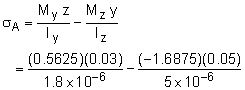| Ch 6. Advanced Beams | Multimedia Engineering Mechanics | ||||||
|
Composite Beams |
Unsymmetric Bending |
||||||
| Unsymmetric Bending | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Stress/Strain |
| 2. Torsion |
| 3. Beam Shr/Moment |
| 4. Beam Stresses |
| 5. Beam Deflections |
| 6. Beam-Advanced |
| 7. Stress Analysis |
| 8. Strain Analysis |
| 9. Columns |
| Appendix |
| Basic Math |
| Units |
| Basic Mechanics Eqs |
| Sections |
| Material Properties |
| Structural Shapes |
| Beam Equations |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
 Cantilever Beam Loaded in Two Directions |
Example |
|
|
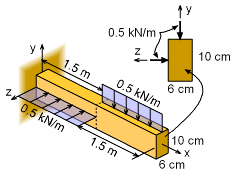
Two distributed line loads act on a cantilever beam as shown in the diagram on the left. Both loads act through the center of the rectangular cross section in the directions shown. What is the maximum absolute bending stress in the wall? |
||
| Solution |
||
|
|
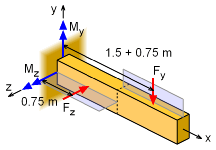
To determine the bending stresses, the maximum moment needs to known. Since this is a cantilevered beam, the maximum bending moment will occur at the fixed end, or the wall. Mz = Fy d = -[(1.5)(0.5)] (1.5 + 0.75) The moment of inertia around each axis is Iz = (0.1)3(0.06)/12 = 5×10-6 m4 |
|
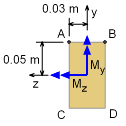 Beam Cross-Section at Wall |
The maximum moment at one of the four corners. From inspection, both the My and Mz moments will cause tension stress in the upper left corner, point A (don't forget that Mz is negative value). Similarly, both moments will cause compression stress at point D. These two points, A and D, will be the maximum tension and conpression stress, respectively. In this case, the magnitudes for both the maximum compression and maximum tension will be equal. Maximum stress at point A, y = 0.05 m, z = 0.03 m, is |
|
Practice Homework and Test problems now available in the 'Eng Mechanics' mobile app
Includes over 500 free problems with complete detailed solutions.
Available at the Google Play Store and Apple App Store.