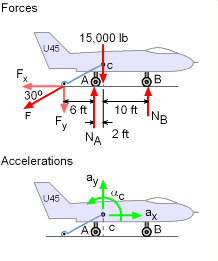
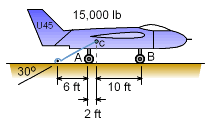
A 15,000 lb training jet is bought to a stop by using an arresting hook. The connecting cable exerts a force F at point C on the plane at an angle of 30 degrees with the horizontal and causes the plane to decelerate at 5g's. The horizontal forces exerted by the landing gears are negligible. Determine the normal reaction forces exerted on the landing gear. The line of the hook passes through the center of mass, point C.

Forces and Accelerates

Both the linear and angular accelerations must equal the forces and moments on the object. The moments are summed about the center of mass, C.

The force exerted by the hook has to counter the horizontal force due to deceleration, 5g. This gives,
   ΣF_x = ma_x
   F cos30 = (15,000/g) (5g)
   F = 86,600 lb

The sum of vertical forces gives,
   ΣF_y = ma_y = 0
   -86,600 sin30 + N_A + N_B - 15,000 = 0
   58,300 = N_A + N_B

The sum of moments about the center of mass gives,
   ΣM_C = I_Cα_C
   -2N_A + 10N_B = 0
   N_A = 5N_B

Solving the two equations for N_A and N_B

N_A = 48,590 lb and N_B = 9,717 lb