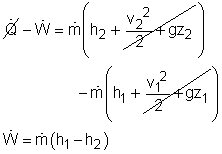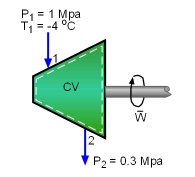
A turbine replaces a throttling valve to reduce the pressure of liquid refrigerant-134a from 1 MPa to 0.3 MPa. The power generated by the turbine and the money saved from this energy conversion need to be determined.

Assumptions:

• The flow is steady.
• The turbine is adiabatic.
• The pressure reduction process is reversible.
• Kinetic and potential energies are negligible.

Schematic of the Turbine

Pressure Reducing process on the
h-s Diagram

Saturated R-134a Temperature Table

Saturated R-134a Pressure Table

Superheated Steam Table

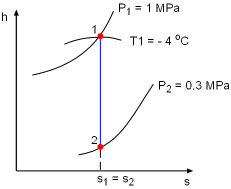
(1) Determine the power generated by the turbine

Take the turbine as a control volume. The inlet is state 1 and the exit is state 2.

Since the assumptions state that the turbine is adiabatic and the process is reversible, this means the process is an isentropic process (will be introduced in the following section). Hence,

      S₁ = S₂

The turbine has only one inlet and one exit. That gives

      s₁ = s₂

where
      s = entropy per unit mass

The saturation temperature of the refrigerant R-134a at 1 MPa is 39.39 ^oC. Hence, the refrigerant R-134a at 1 MPa and -4^oC is a compressed liquid. In the absence of compressed liquid data, the properties of compressed liquid can be approximated by the saturated liquid data. At -4^oC, the saturated properties of refrigerant R-134 are

      P_sat = 0.25274 MPa
      v_f = 0.0007644 m³/kg
      h_f = 44.75 kJ/kg
      s = 0.1777 kJ/(kg-K)

The properties of state 1 are:

      h₁ = 44.75 + 0.0007644( 1- 0.25274)(10³)
           = 45.32 kJ/kg
      s₁ = 0.1777 kJ/(kg-K)
      v₁ = 0.0007644 m³/kg

At state 2, the pressure and the entropy are known, hence, enthalpy of state 2 can be determined as

      h₂ = 44.75 + 0.0007644( 0.3- 0.25274)(10³)
          = 44.75 kJ/kg

The energy balance of the turbine is:

substituting the mass flow rate and the enthalpies into the above equation yields

       = 200(45.32-44.75) = 114 kW

(2) Determine how much the turbine can save the facility per year

If the turbine operate 8,760 hour per year, the amount of power produced per year is

      W_yr = 114( 8760) = 998,640 kWh/yr

If the price of the electricity is $0.08/kWh, then 998,640 kWh of electricity is worth of

      998,640 (0.08) = $79,891

The facility can save $79,891 if the throttling valve is replaced by a turbine.