| Ch 6. Structures | Multimedia Engineering Statics | ||||||
| 2-D Trusses: Joints | 2-D Trusses: Sections | 3-D Trusses | Frames and Machines | ||||
| 2-D Trusses: Method of Sections | Case Intro | Theory | Case Solution | Example |
| Chapter |
| 1. Basics |
| 2. Vectors |
| 3. Forces |
| 4. Moments |
| 5. Rigid Bodies |
| 6. Structures |
| 7. Centroids/Inertia |
| 8. Internal Loads |
| 9. Friction |
| 10. Work & Energy |
| Appendix |
| Basic Math |
| Units |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
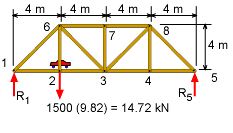
| Solve for Truss Reactions |
||
|
|
The force in the top member 6-7 needs to be determined when the 1,500 kg car is at joint 2. First, solve for reactions R1 and R5 by applying
both the moment and force equilibrium equations for the whole truss. ΣFy
= 0 |
|
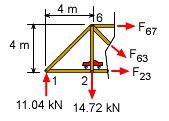
| Cut Truss at Required Member |
||
|
|
Since, the member 6-7 needs to be determined, that member must be included in the cut. However, there is is usually more than one location to make a cut. It is easiest to make a cut where only three or less members are cut. Thus, a vertical cut through members 6-7, 6-3 and 2-3 was done as shown at the left. Notice that there are only three unknowns, which can be solved for with the three equilibrium equations. ΣFy = 0 ΣM1
= 0 For this cut location, you need to use at least two of the three equilibrium equations. The number of equations may be reduced by other cut locations. |
|
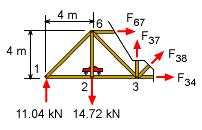
| Alternate Cut |
||
|
|
By carefully choosing where the cut is made, the number of calculations can be reduced. For example, if a cut is made through members 6-7, 3-7, 3-8, and 3-4 it is possible to solve for the member force F67 with one equilibrium equation even though four members are cut. Since all unknowns, except F67, go through ΣM3
= 0 The solution is identical to the solution for the previous cut. |
|